7
$\begingroup$

At the present time, about 70% of the earth's surface is liquid water (perhaps that includes floating ice around Antarctica, and the Arctic Ice cap).

What percentage of the Earth's surface would be water if ALL the ice had melted?

I've seen illustrations of the new shoreline, and I've seen estimates of the rise in sea level, but no answer to my question.

Improve this question
$\endgroup$
7
  • 1
    $\begingroup$ An accurate calculation would need to consider post-glacial rebound. $\endgroup$
    – gerrit ♦
    Commented Nov 28, 2018 at 10:38
  • $\begingroup$ I'm just interested in a blurry approximation. $\endgroup$
    – Jim Brenneman
    Commented Nov 29, 2018 at 20:33
  • $\begingroup$ earthscience.stackexchange.com/questions/7228/… $\endgroup$
    – Keith McClary
    Commented Nov 30, 2018 at 21:31
  • 2
    $\begingroup$ keep in mind melting ice is not the only thing causing sea level rise, thermal expansion of the ocean water accounts for far more rise. $\endgroup$
    – John
    Commented Dec 31, 2021 at 3:17
  • $\begingroup$ Ice does not obey the law of archemedes, it is buoyant, it is also an exception to the rule of thermal expansion as it expands when frozen thereby taking up more space when frozen. Liquid molecules are more densely packed than gaseous molecules and less densely packed than solid matters molecules. Water also always flows to the lowest point which to me is the compounds most prevalent characteristic. In doing this it creates things we know called stalactites and stalacmites and underneath every inch of surface on this planet we have a thing called a water table, i think the answer to this quest $\endgroup$
    – Mark Lawlor
    Commented Apr 4, 2022 at 20:12

1 Answer 1

Reset to default
7
$\begingroup$

As @gerrit commented, a precise calculation would need to incorporate a litospheric model to account for isostatic post-glacial rebound. But the "blurry approximation" you want, can be obtained based only on topographic data. This approximation would be reasonably accurate if the melting of the ice happens quickly.

For this, we need topographic data of the current surface, and of the bedrock topography. That's the bare ground below the ice. The only source of such data at a global scale (at least that I'm aware of) is ETOPO1, at a resolution of 1 arc-minute (roughly 1.8 km).

A visualization of current surface data from ETOPO1, using an equal-area projection (Eckert IV) and setting the sea level at 0 m looks like this:

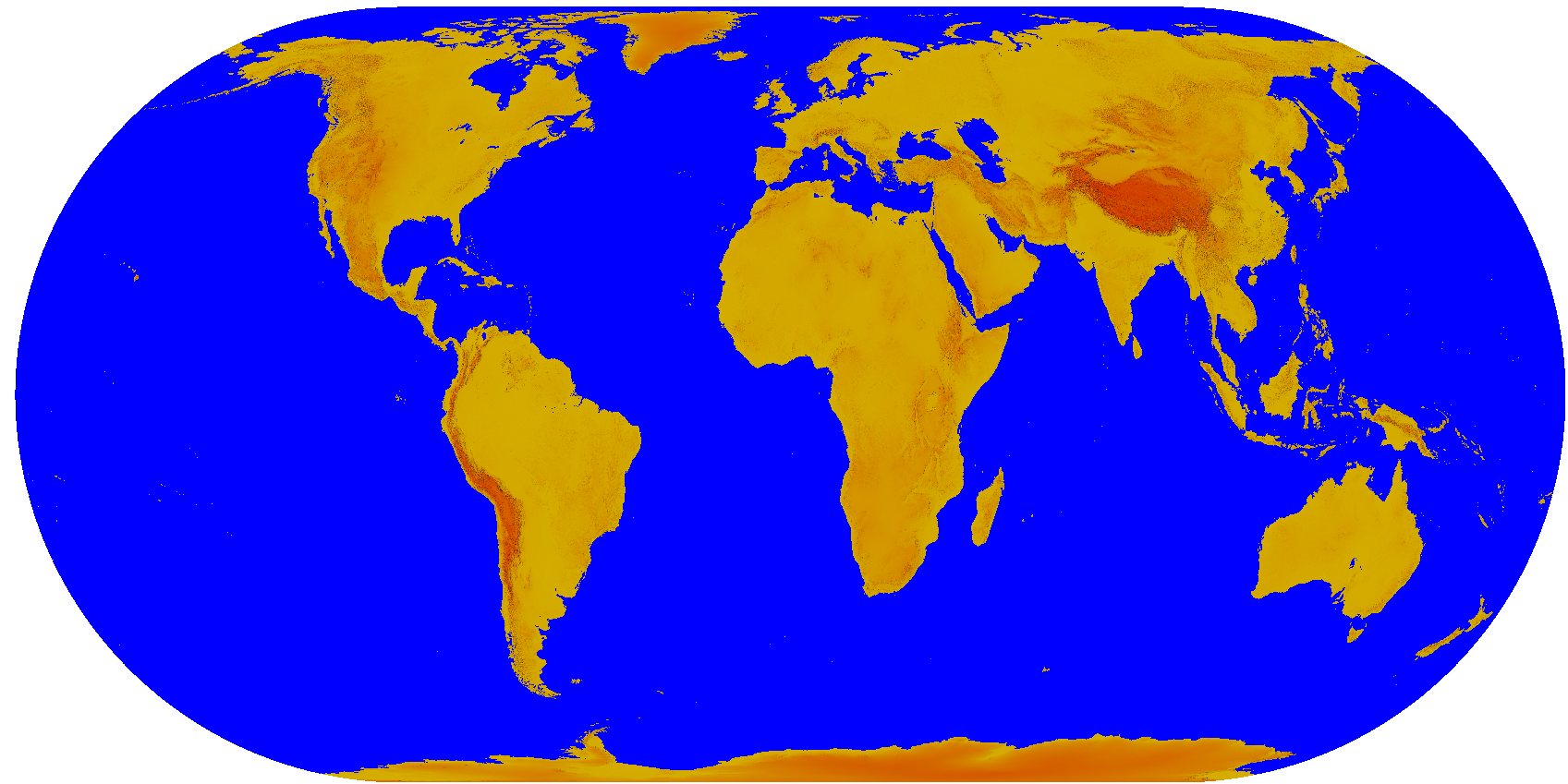
enter image description here

And if we measure sea and land areas, it says that currently, a 70.97% of the global surface corresponds to oceans, pretty consistent with the figure you have. Be aware that this simple approach does not consider lakes or rivers, only oceans. It would also misinterpret below sea level dry land as ocean. However, all those factors are arguably minor contributions at a global scale.

Now, if we use the bedrock version of ETOPO1, and set the sea level at 66.5 m, consistent with the best estimates of water stored in land ice. The globe would look like this

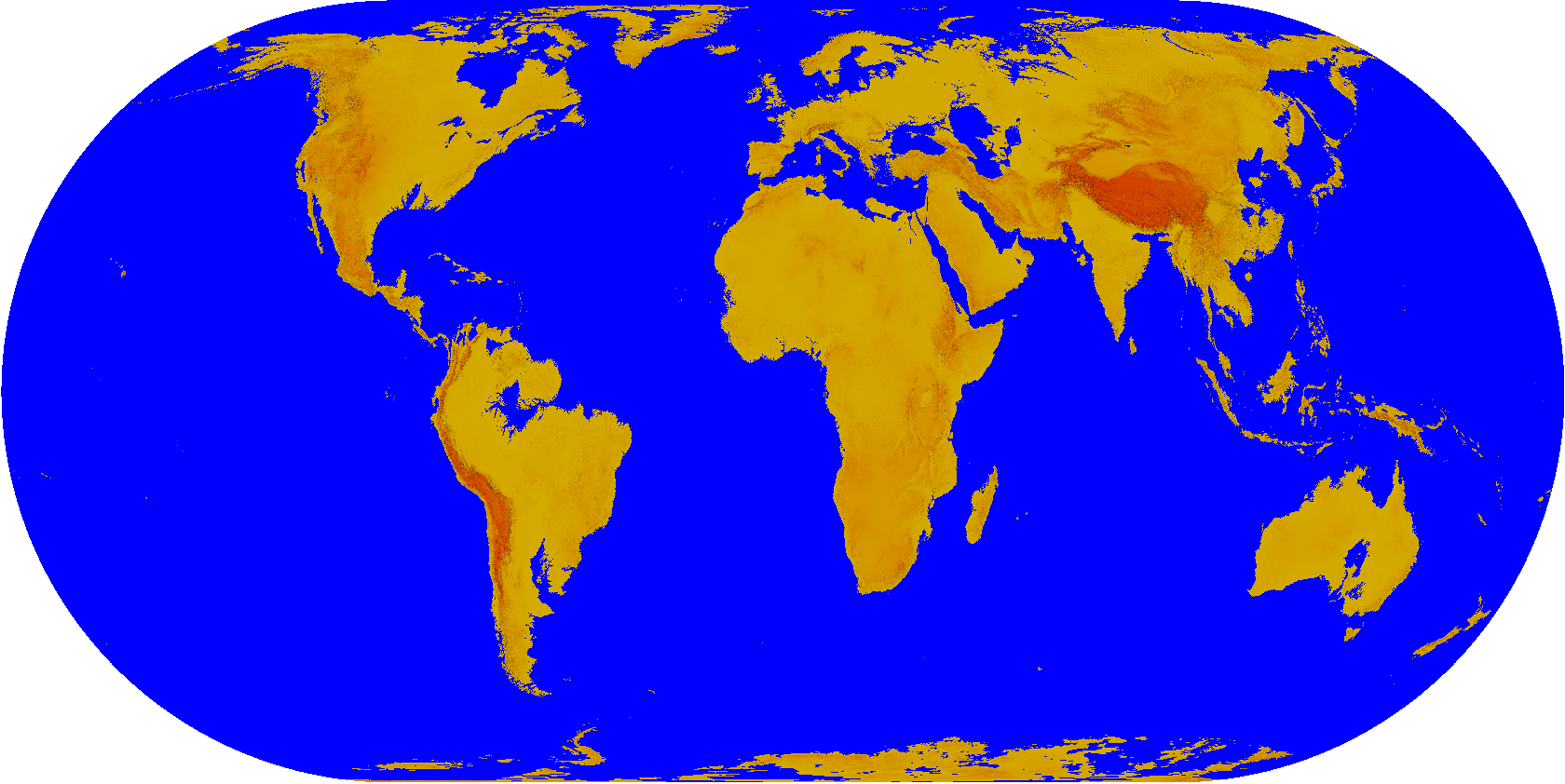
enter image description here

In this case, if all the ice melts, the fraction of the Earth's surface covered by water would be 75.18%. Equivalent to an increase of 4.21% relative to the current value.

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged or ask your own question.