Why is Earth's outer-core liquid?

Question

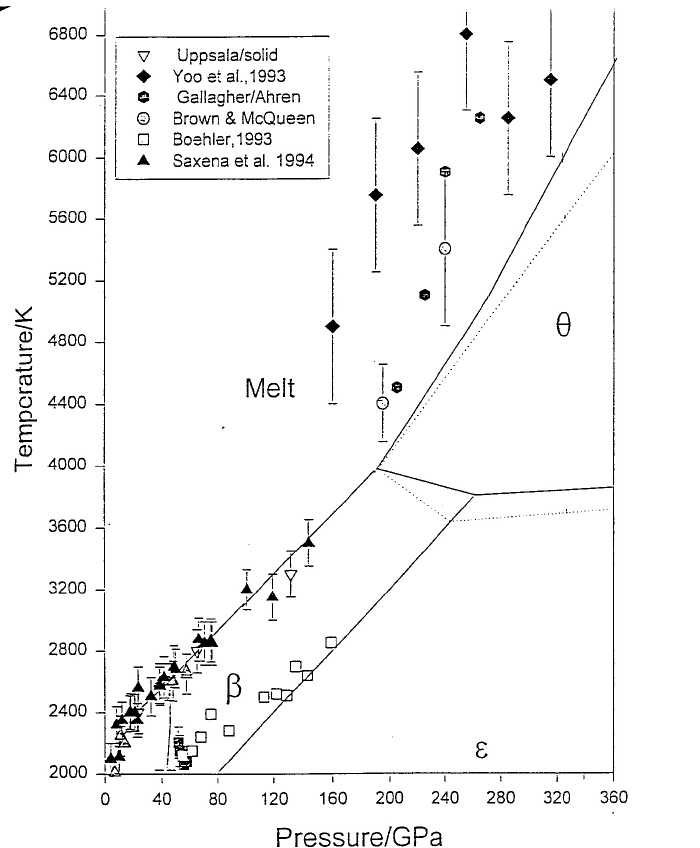
The Earth's inner core is solid because despite the enormous temperature in this region, there is also enormous pressure there, which in turn raises the melting point of iron and nickel to a value above the Earth's core temperature.

Now as we move out from the solid inner core, temperature drops, and pressure also decreases. Obviously because the inner core is solid but the outer core is liquid, we must conclude that the drop in temperature vs the drop in pressure must be lower than the gradient of 16 degrees/GPa shown in the diagram below (link to source), given that at the outer-core temperature has exceeded the melting point of iron/nickel, which is a function of pressure.

In other words, the drop in pressure must be quite significant compared to the drop in temperature as radius increases from the core.

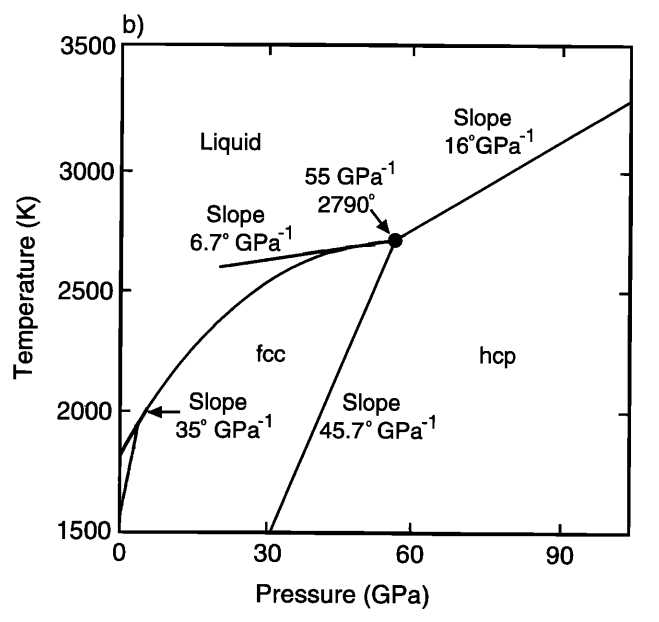
So how is it that pressure drops off fast enough relative to temperature to give rise to the liquid outer-core. A good answer will explain how temperature drops off with radius and how pressure drops off with radius and how these compare to give rise to the liquid outer-core.

Answer 1

First, you need a phase diagram that goes to higher pressure. The pressure at the inner/outer core boundary is over 300 GPa. The one in the question would only get us into the mantle:

(link to source)

A typical temperature and pressure at the outermost part of the core would be 3750K and 135GPa, which is in the liquid region of the phase diagram.

For more data on pressure and temperature as a function of depth see this University of Arizona source. All appropriate credit to Marcus Origlieri.

Answer 2

The pressure gradient is given by hydrostatic equilibrium. In a solid, this may not be exactly true, but creep will make it so. Let $p$ be the local pressure, $g$ be the local acceleration of gravity and $\rho$ the local density. Imagine a small element of volume with area $A$ horizontal and height $\Delta h$. Its mass is $\rho A \Delta h$ and it is attracted downward by force $g\rho \Delta h$ This has to be balanced by the pressure difference between the top and bottom, so $\frac {dp}{dh}=g\rho$. $g$ can be determined (assuming spherical symmetry) by just counting the total mass at smaller radii.

Answer 3

Just to add to Dave's answer, the above phase diagrams give the illusion of high precision. In fact, in addition to the temperature distribution, we are not even entirely sure how many sub-solidus phases there are. See for example, Ahrens et al: http://web.gps.caltech.edu/~sue/TJA_LindhurstLabWebsite/ListPublications/Papers_pdf/Seismo_2069.pdf Moreover I was rightly corrected, in a previous answer, when I asserted that the core composition is Ni-Fe with a large sulphur impurity. In fact, although I was probably correct, there is plenty of scope for S2, Ni and other heavy metals, H2, OH-, and other impurities in the core, at unknown concentrations, most of which can create a eutectic depression of the solidus line - that is, shifting the liquid-solid boundary down-temperature.

Answer 4

Though both the inner and outer core are made of the same material, the melting point of inner core is increased due to the increased pressure, so the inner core is in a solid state and outer is a liquid .