4
$\begingroup$

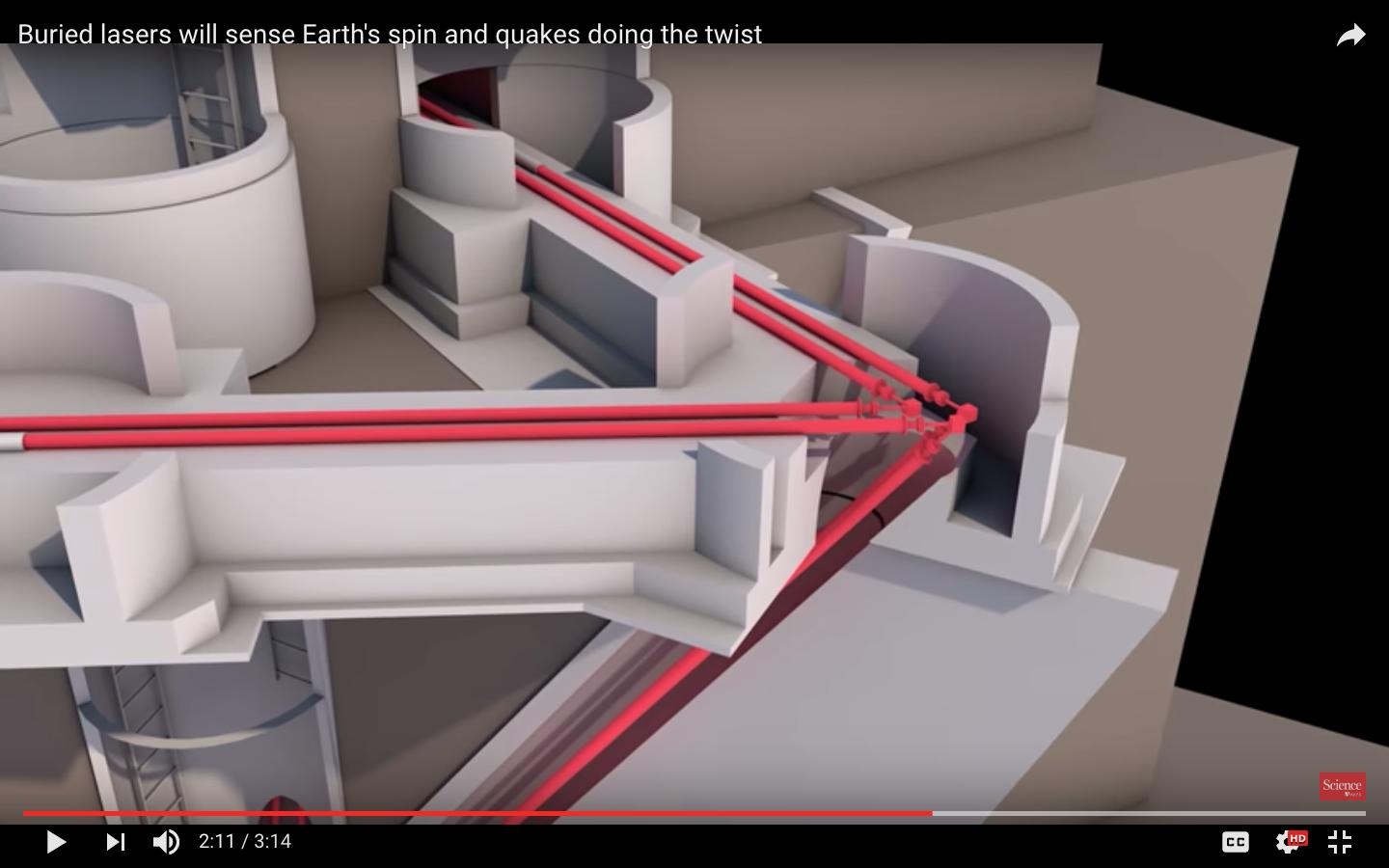
The Rotational Motions in Seismology (ROMY) instrument is a giant laser gyroscope used to generate extremely precise 3D rotation measurements of planet Earth. However at the end of the video below they mention that "seismologists want to..." use small laser gyros to measure seismic tilt motion as part of earthquake monitoring.

Is there a lot of interest in this? Have compelling questions been identified that could be address by adding tilt motion to translational motion monitoring, or is it a case of nobody's looked, there might be something interesting there, better find out?

https://youtu.be/MXYV6wNdZm8?t=102

enter image description here

Improve this question
$\endgroup$

1 Answer 1

Reset to default
3
$\begingroup$

The intent with this (very, very cool) device seems to simply be to improve hazard assessment and mitigation by providing higher resolution motion data.

It reminds me of tiltmeters used on volcanoes. These devices measure changes in the flank slope of a volcano in order to assess volatility and risk (how the magma chamber is developing, etc.).

This kind of technology isn't new, but it's only been available in a precious few places. The ROMY will be able to cover a wide area, but the real holy grail is to have many mobile stations that can record local movements worldwide (as they mention in the video).

Improve this answer
$\endgroup$
2
  • $\begingroup$ The primary purpose of ROMY is for detecting changes in the rotation and tilt of the Earth, which can be affected by all kinds of geologic and surface phenomenon - things that move mass around. The difference between this kind of gyroscope and a tilt meter is that the gyroscope measures rotation rate, so it becomes very sensitive to fast tilting events like those that might be in seismic waves. Theoretically they could measure gradual tilting, but real-world limitations of noise within the lasers makes that more challenging. I had no idea that tiltmeters were used near volcanos though! $\endgroup$
    – uhoh
    Apr 27, 2017 at 19:37
  • $\begingroup$ I've asked this follow-up question. $\endgroup$
    – uhoh
    Apr 27, 2017 at 19:45

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge that you have read and understand our privacy policy and code of conduct.

Not the answer you're looking for? Browse other questions tagged or ask your own question.