Considering how old the Antarctic ice cover is, why isn't it much thicker?

Question

The Wikipedia article on the subject of the "Antarctic ice sheet" says that:

The icing of Antarctica began in the middle Eocene about 45.5 million years ago and escalated during the Eocene–Oligocene extinction event about 34 million years ago.

The article later says that:

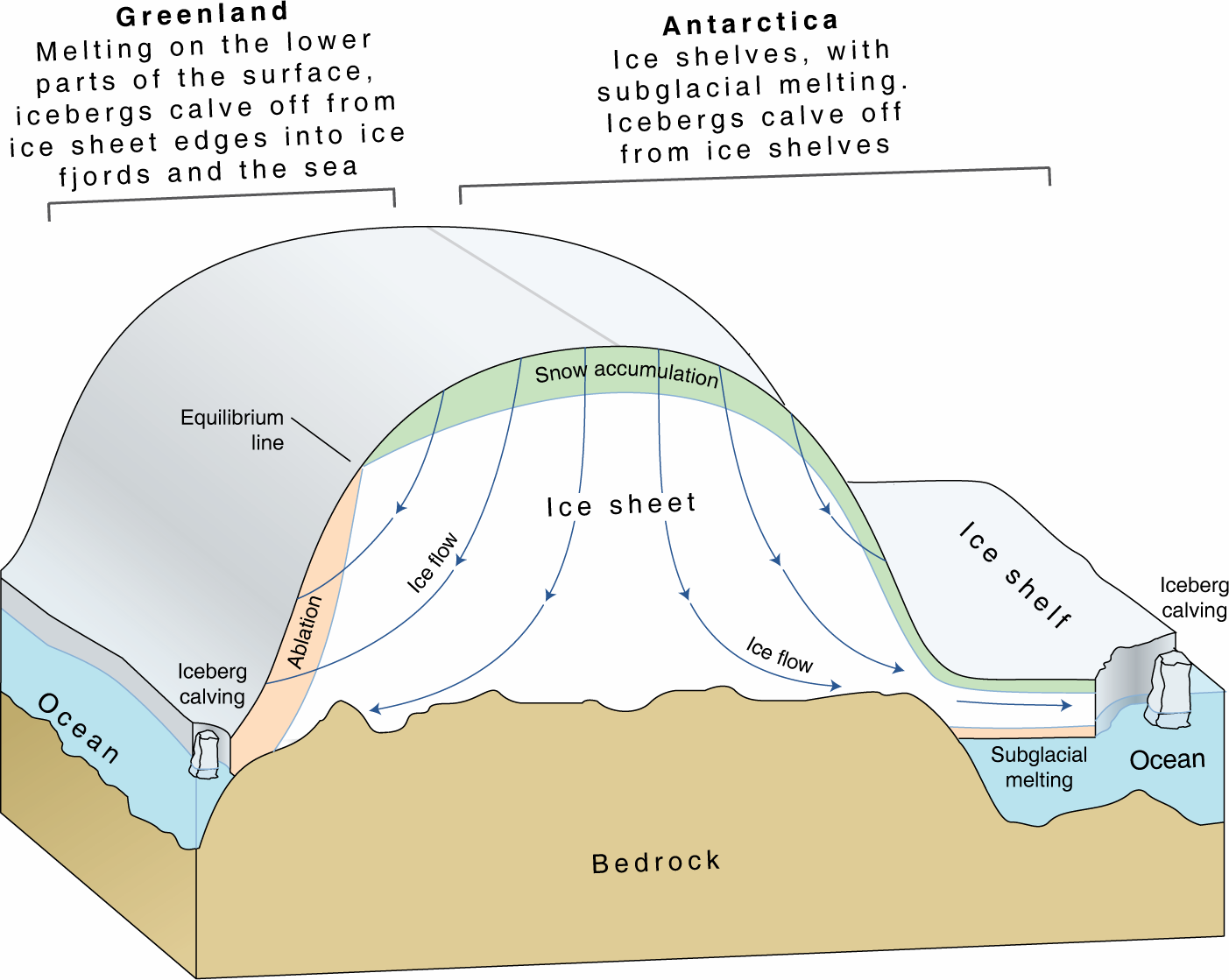
Ice enters the sheet through precipitation as snow. This snow is then compacted to form glacier ice which moves under gravity towards the coast.

However it looks like, from the photos of Antarctica, that this transfer of ice to the coasts is not happening everywhere on Antarctica. Rather, many places seem to be under a perpetual ancient ice cover.

If the precipitation was recycled at a continuous rate everywhere, it shouldn't be possible to obtain a 1.5 million year old core sample (according to an article in Nature) or structures such as Vostok Station that covers an ancient lake.

A similar location is Dome F that according to Wikipedia has a yearly precipitation of about 25 mm (millimeters; approx. 0.98 inches).

So the question is, if we have a 25 mm precipitation per year for even just 10 million years, we should have an ice cover that's 250 kilometers thick. Or if we had it during just a 5 million year time span, it should give us an ice cover that's 125 kilometers thick.

Reasonably we could allow for some compression of the ice but still keeping in mind that we are not talking about a neutron star here.

How come the current Antarctic ice cover is just a couple of miles thick?

Answer 1

Ice floats with gravity towards lower elevation, the flow continues until the base of the ice sheet becomes floating and the ice forms an ice shelf or icebergs.

Due to the subglacial topography, basal melting and mass balance, the flow velocities vary over a large range, faster outflows are glaciers. The pattern is somehow similar to how rivers transport rainwater towards the coasts.

The thickness of the ice sheet is controlled by a complex relation between accumulation, ablation and compaction.

Flow velocities from MEaSUREs (NASA), plotted from Quantarctica

The ice velocity map shows, that some areas are unaffected by the flow towards the coast. Some of these areas are ice-free. Also at some inland locations, as Vostok, the velocity is very slow. Also, note that this is the surface velocity. At the base, the ice flows slower or not at all.

To investigate the mass balance further, I recommended you to download Quantarctica and look closer at the datasets. Shepherd et al (2012) is a good first read about the methods used to estimate changes in ice mass and the present changes.

Answer 2

An interesting point to consider comes from your assumption of a constant rate of precipitation. Many earth system processes have non-trivial stochastic variations (or high-dimensional chaos). Consider the conservation of mass for ice:

change in ice thickness = (precipitation, mass in) - (pick your favorite process, mass out).

Both (mass in) and (mass out) processes have significant random variability, both in space and time. When you integrate a random variable, a special thing happens: you get a random walk. The figure below shows 5 random walks

_{(source: jburkardt at people.sc.fsu.edu)}

Notice how several different random walks spread apart with time? A random walk has the property that, the standard deviation of all walks together, grows with the square root of time. As in, the spread of all positions at a given point in time, grows proportionally to the square root of time. If the random walk wasn't random, but was constant instead, you would have a straight line (this is the case you described).

So here is the first point to be made: a constant rate of precipitation leads to a linear increase in ice thickness, growing without bound. By adding in some randomness to approximate nature better, the rate at which the ice thickness grows is fractional with time rather than linear. A true random walk is still unbounded, and so there are clearly some negative feedback mechanisms at play as mentioned in the previous posts.

Second point (actually answering your question). As this is a 2d surface, you could model glacial topography as 2D random field. In the absence of forcing, the dynamics of glacial topography is effectively a diffusion equation. A randomly forced diffusive surface has a natural (or internal) cutoff length scale, or scale above which ice won't grow. However, this cutoff scale would be a function of ice surface diffusivity, rather than a feedback mechanism between the system and forcing. So this is all to say, it could be a feedback mechanism, or it could be set internally by the material properties of the system. I'll look and see if anyone has written papers on this yet.

The figure below shows an example of a 2D random field. I don't know how exactly it was generated, but it's basically the kind of solution you would get from a 2D diffusion equation with random forcing.

Answer 3

There is a balance between precipitation on the one hand, and sublimation and outflow on the other. Ice evaporates directly to water vapor, especially during the summers. The thicker the ice, the greater the pressure at the bottom of the ice owing to the weight of the overlying ice, and hence the faster the outflow. The thicker the ice, the less the precipitation, because the less the atmosphere above the ice sheet. The pressure at the bottom of an ice sheet 250 km thick, or even 125 km thick, would be so great that outflow would be very much faster than at present, and very much exceed even current average precipitation of perhaps 1 inch per year. Indeed precipitation would be ZERO for such a thick ice sheet because its height would be above any appreciable atmosphere. Even neglecting sublimation and outflow, an ice sheet could never attain a thickness of 125 km, much less 250 km, because precipitation over the ice sheet would vanish well before such a thickness was attained.

Answer 4

According to "Ice Sheet Modeling"

ice behaves as a deformable plastic material, which means that there is a critical shear stress, below which no strain (deformation or flow) will occur ... If the slope is too low, the basal shear stress will not match the critical shear stress, ... but as snow piles up, ... flow will begin. ... The result of this is that a glacier has an equilibrium profile

A simplified model based on this theory results in the height growing approximately as the square root of the distance from the edge.

Snow falling on the surface does not stay on the surface, but takes a deeper journey through the ice sheet as it is buried by subsequent snow and is compressed into solid ice. Snow falling in the deepest interior parts of Antarctica can take over 100,000 years to reach the ocean.
NASA