I gave this one some thought and ran some numbers.
The real answer to your question is that you would be lighter because the material that makes up continental plates is lighter than the basalt rock under it. It might not look that way but continents, mountains and oceans are actually balanced with about equal weight under them. Continents are above sea level because of larger amounts of crust which is lighter than basalt. The earth, which is pretty malleable as a whole, adjusts for significant unbalanced changes in mass, it's still rebounding from the melted glaciers from the last ice age for example.
This happens with Mountains too, also slowly, but mountains are formed over millions of years so the adjustment has time to take place. Mountains are formed when two continental plates push against each other, one slides under the other, so both what forms the mountain, and what goes under the mountain is lighter than basalt under the Earth's crust. When you climb a mountain, you're not actually on top of more mass because much of the rock under you is lighter rock. Mountains are actually puffy (I always wanted to say that). If mountains were actually greater mass relative to the (relatively) spherical Earth, then they'd sink into the Earth, which they do to some extent, but, as I said above, Mountains are puffy buoyant compared to the Earth's crust, so the mass is pretty much the same on top of the mountain and at the foot of the mountain.
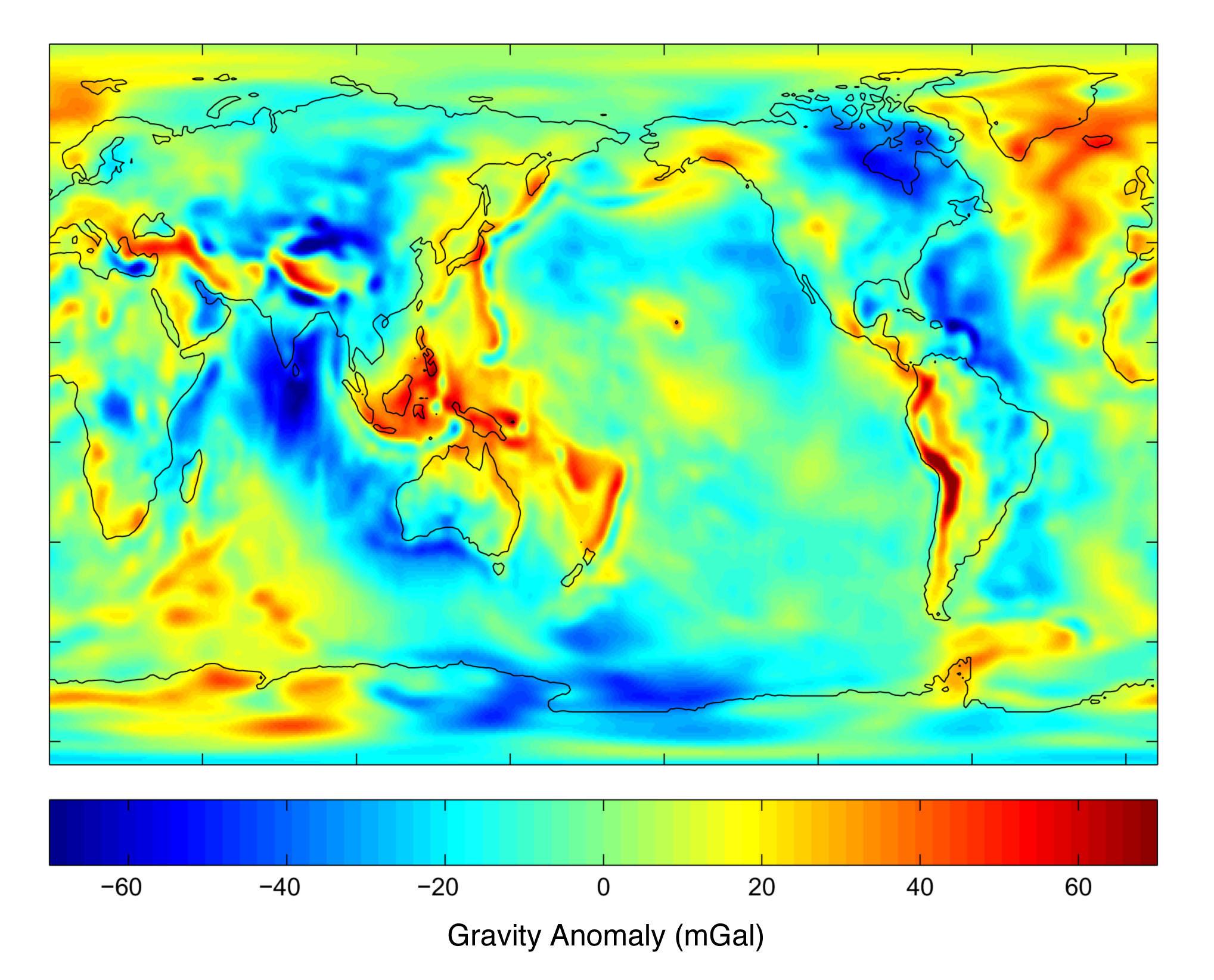
That's not to say the Earth is perfectly balanced, it's definitely not, but the imbalance doesn't appear to follow mountain ranges. Google "Earth Gravity Map" for more on that. Article with pretty picture here.
But I suspect, your question wasn't just real world but also a mathematical query, so, moving on. If we create a cone shaped mountain, a large flat one, lets say it's 6.371 km tall (reason I picked that number will become clear soon), and lets give it 4 times that as a radius or 25.484 km radius to it's base and a specific density of 2.7, the mass of our mountain, using 1/3 base times height is: 3.1416 * .333333 * 2.7 * 25,484^2 * 6,371 * 1000 (the 1,000 is for density of 1,000 KG per cubic meter at specific density of 1) = about 1.17x10^16 KG.
So, on top of this artificial mountain that, if it was actually added to the earth, it would push the earth down and the earth would adjust it's mass around it over time, but ignoring that, the mountain is 1/1000th the radius of the earth so the distance from the Earth's center is increased by 0.1%
The mass of the mountain, 1.17E16kg is about 1/510,000,000 the mass of the Earth, 5.97E24kg, So we have a mass increase of about 0.0000002% and a corresponding reduction in gravitation by increased distance to the center of the earth (the square of 0.1%), or 0.0001. or, 510 times as much is lost by the higher altitude than is gained by the increase in mass. Now if you make the mountain flat enough, you'd probably be able to increase gravity the tiniest bit, but past a certain point the foot of the mountain is so spread out that the calculation grows inaccurate. The short answer is simply, no, as the other two posters have said. The mass of any mountain is simply insufficient to increase the force of gravity more than it loses by what's lost in the higher altitude.
A 3rd real factor, completely unrelated to your question is air density. Heavier air does create some buoyancy which reduces our weight and the gravity we feel, this effect varies with high and low pressure and temperature, but on an average day at sea level air buoyancy reduces our mass by about .12%, so if you weighed yourself in a vacuum chamber vs your regular bathroom routine, you'd find you weigh about 2 or 3 ounces more for an average person. Climb a mountain, the thinner air, you might gain an ounce due to loss of air buoyancy and that more than makes up for any small changes in gravity, so if you measure gravity by a scale and a weight, things weigh more on top of a mountain, because of thinner air, even though the G force is slightly lower.
The rotation of the earth and the centrifugal force also decreases mass, depending on how close the mountain is to the equator, (see picture). as does the bulge of the Earth around it's equator, but all these changes are tiny.