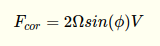David's answer is comprehensive, but perhaps a little technical for the level of the question, so I'll try to offer another angle.
Coriolis is an apparent force that causes an air parcel to curve to the right in the northern hemisphere and to the left in the SH. It and can be explainedunderstood by a conservation of angular momentum. With regards to how Earth's rotation effectsaffects the apparent horizontal movement of wind, these figure gives a nice summary:
We can see that closer to the poles, an aircraft will appear to have more Earth "move" beneath them while in the air. Another way to visualize the effects of Earth's rotation is imagining a person standing at the equator and at the pole. Equator-person will feel no rotation along their vertical axis with respect to the planet, while pole-person will continuously spin in place with respect to the planet's axis.
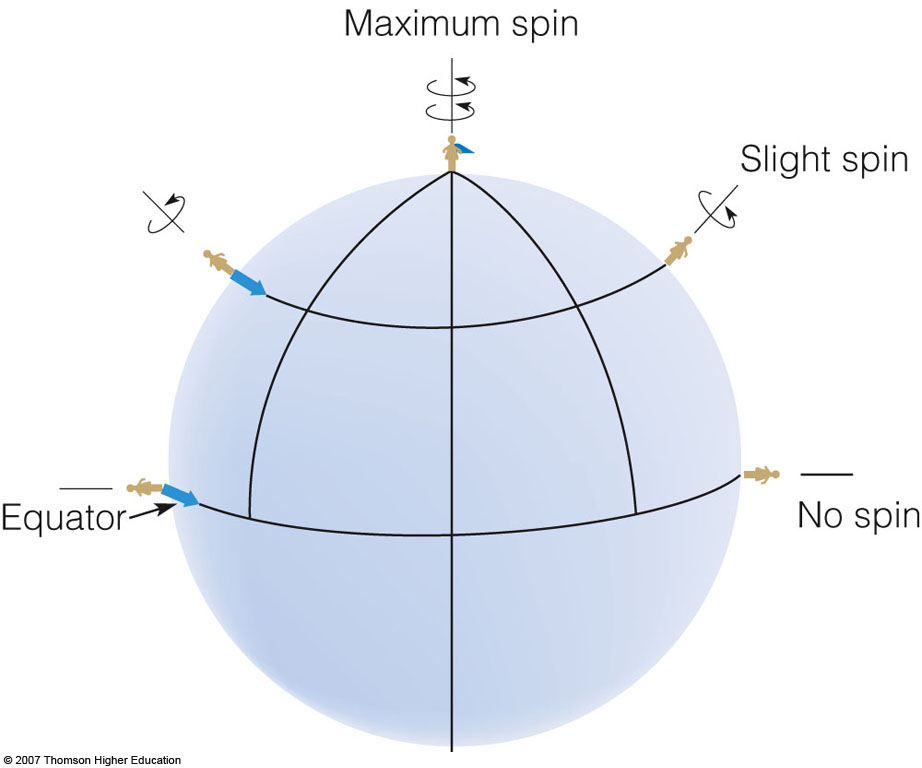 Because air parcels are in constant motion over a rotating surface, the amount of apparent horizontal deflection is determined by the latitude of the air.
Because air parcels are in constant motion over a rotating surface, the amount of apparent horizontal deflection is determined by the latitude of the air.
A simplified version of north/south Coriolis force operating on horizontal winds is given by:
Where omega (Ω) is the rate of earth's rotation, or angular velocity, (7.292 x 10-5 sec-1), and phi (ϕ) is the latitude of the wind velocity component (V) in question. Because Earth spins counter-clockwise, the apparent deflection of wind is 90° to the right in the northern hemisphere and 90° to the left in the SH.
From the equation we can see that if the latitude is zero, then the horizontal north-south deflection contribution of Coriolis is zero. It's important to note that Coriolis has more components than north-south contributions, like in the vertical and east-west, but for the purposes of wrapping your head around the basics this should suffice.