If we consider that by "Assume daily cycle and convection and so on operate as usual" you meant that all heat transport from/to the pole remain as it is today. Then, we can do a back of the envelope calculation. This calculation will at least give you an order-of-magnitude answer, and we can then consider everything that would affect the result.
Currently both poles radiate more energy than they receive trough direct solar irradiation. This is because they are colder than the rest of the planet. Therefore, there is a neat heat transport from the equatorial latitudes to the poles. That heat travels on atmospheric and oceanic currents. This figure nicely summarize this radiation balance:
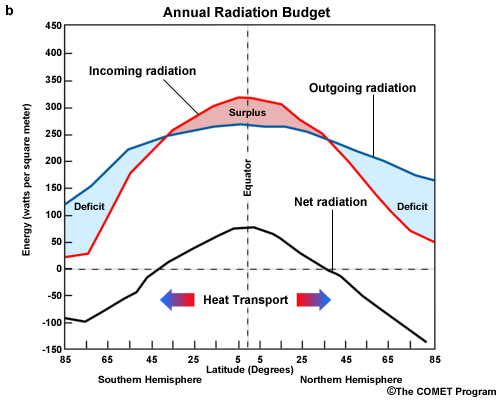
Figure from here ©The COMET Program
If we consider that the heat transport depicted at the bottom of the figure remains the same. The only change in the energy budget would be from solar irradiation, and once they start warming the only way to release the additional heat and reach equilibrium would be trough infrared radiation (black body radiation).
The poles receive zero energy on the equinox and the winter solstice and 12.64 kWh/m$^2$ per day on the summer solstice. Therefore a rough yearly average would be 3.16 kWh/m$^2$ per day. So, if we were locked on a summer solstice state you would receive four time more energy, and none if locked in a winter solstice state.
Summer solstice locked pole
Let's consider the summer solstice case first. If we call $E_1$ the current amount of outgoing radiation from the pole, and $T_1$ the current temperature. The Stefan–Boltzmann law states that
$E_1 = \sigma T_1^4$
Analogously, if $E_2$ and $T_2$ represent the summer solstice locked state, you have
$E_2 = \sigma T_2^4$
Considering that $E_2 = 4 E_1$
You can write that
$4 = \frac{T_2^4}{T_1^4}$
Or
$T_2 = (4)^{1/4}\ T_1 = \sqrt{2}\ T_1 \approx 1.41\, T_1$
Therefore the temperature would increase 41%. It doesn't sounds much, but considering that the temperatures above are in Kelvin, it es a lot! In fact, with the current mean temperature in the north pole of -16 °C (257K), it would go up to +89°C!! And the South pole would go from the current -49°C (224K) to +42°C.
This diverges from any posible reality because with 89°C at the north pole there would not be a net influx of energy from the equatorial latitudes as we are assuming. Instead, atmospheric and oceanic currents would take a lot of that heat down south and the temperature would equilibrarte at a much lower value.
Note that in the above calculation I've ignored the energy flux due to advection in atmospheric/oceanic currents. I did so because that flux is about five time smaller than the flux from solar radiation, so it won't change much the results and ignoring it simplifies the math. You can do the calculation considering those flows if you want.
Winter solstice locked pole
In the case of a pole locked to a winter solstice. There would be no incoming solar radiation at all. Therefore, all incoming energy would be transported by currents from equatorial latitudes. In that way, we can see in the above figure that the north pole receives about 150 W/m$^2$, and the south pole about 100 W/m$^2$. The equilibrium temperature would be reached when they radiate the same amount. So, using Stefan–Boltzmann law again we can do some rough calculations.
Let's call the south pole temperature $T_{SP}$ and $T_{NP}$ for the north pole. Then:
$T_{SP} = \left(\frac{E_{SP}}{\sigma}\right)^{1/4} = \left(\frac{100 \,W/m^2}{5.67\times 10^{-8}}\right)^{1/4} = 205 K= -68°C$
And for the north pole
$T_{NP} = \left(\frac{E_{NP}}{\sigma}\right)^{1/4} = \left(\frac{150 \,W/m^2}{5.67\times 10^{-8}}\right)^{1/4} = 227 K= -46°C$
But again, with such cold temperatures the actual inflow of energy would be grater and the equilibrium temperature would not be that extreme.
In any case, that condition would be favourable to the growth of a massive ice sheet in the corresponding polar area, in the same way I describe in this question and answer.
As a final thought. For this condition to happen in real life you would need something analogous to Tidal locking to lock the period of Earth's precession (currently about 26,000 years) to exactly one year. And don't know of any mechanism that could achieve that.
