Does any mineral crystallize in the shape of an oblique square prism? If so, what crystal system (monoclinic, cubic, hexagonal, etc.) would such a mineral fall under?
2
-
$\begingroup$ Can you clarify what you mean by "oblique square prism"? Also, when you say "shape", are you referring to the unit cell or a more macroscopic property like crystal habit? $\endgroup$– g.z.Commented Mar 6, 2019 at 20:33
-
$\begingroup$ I was referring to the macroscopic crystal habit. What I meant was that two axes are the same length and at right angles to each other, and a third axis is a different length and oblique (not perpendicular) to the other two axes $\endgroup$– resplaineCommented Mar 7, 2019 at 22:25
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
If "oblique square prism" is this, where the top and bottom planes are parallel, then yes, there are. Carbonates (members of the Calcite and Dolomite, but not Aragonite, groups) have perfect cleavages that leave them with such habit. But be warned that they do not crystallize with such habit, it is a product of an effort that forced it to break accordingly to its cleavages.
A beautifully euhedral calcite crystal.
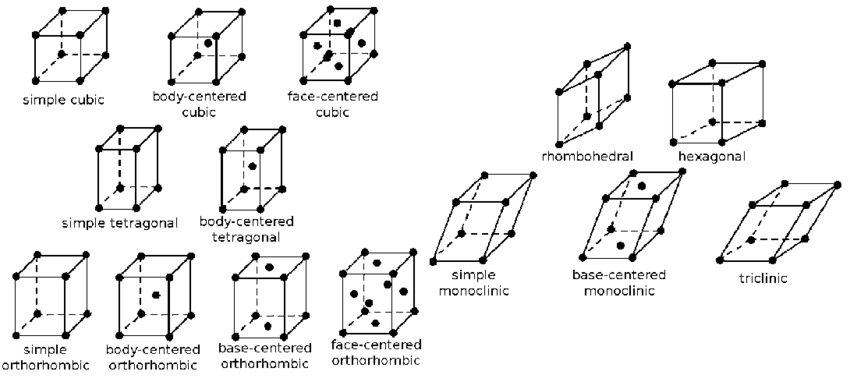
If, however, you're looking for a mineral which crystallizes in such shape, then the answer is no. There are 14 Bravais lattices (to put it simply, a Bravais lattice is the atomic arrangement that makes the smallest unit of a crystal, and so a crystal is a repetition of it). Each vary on edge ('line') length and angular relationship and obey some rules which are irrelevant for this discussion. The point is, none of the Bravais lattices has the edge parameters a, b and c (thickness/depth, width and height edges, respectively) in equal sizes (i.e. a = b = c) and angles different than 90 degrees between them. Which means that no crystal will ever be fundamentally crystallized in such a form.
The 14 Bravais lattices. Any solid must obrigatorily crystallize in one of these 14 lattices. It's a natural rule.
