stretch phenomena after NMO application on seismic data

Question

after the NMO application we have stretch for far offset , i read a lot of articles but i can't have a complete idea how this phenomena is really created ! any one have a rich explanation ?

Answer 1

My understanding is the following (NB: it could be wrong!).

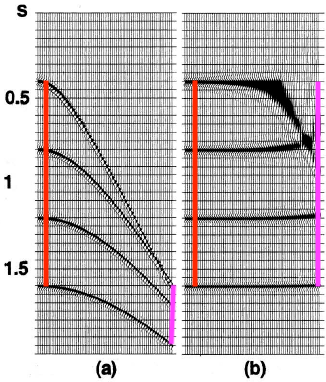
1. The assumption is that on a common shot gather, your travel time follows a hyperbolic curve: $$ f(x)=t^2=t_0^2 + \frac{x^2}{v^2}, $$ where $t_0$ is the zero-offset travel time, $x$ the offset and $v$ the speed of the medium above the interface. The hyperbolic travel-times, for example, create gathers like the one shown in https://wiki.seg.org/wiki/NMO_stretching#/media/File:Ch03_fig1-9.png (which is figure '(a)' below).

2. Furthermore, the recorded wavelet has the same shape along the time ($t$) direction, regardless of which offset ($x$) you recorded it. Hence, on the figure below, check that there is no discernible change in the wavelet shape in figure '(a)' below as you move along the hyperbolic curve.

3. Then, NMO correction essentially comes down to stretching the original data. In the figure '(a)' below, I put a red line close to the zero offset point, and a purple line at far offset. In figure '(b)', which is an NMO-corrected picture, I highlight that same lines, with identical colors. You see that the zero offset (red line) hardly requires a change, but the far offset (purple line) needs to be stretched considerably to create a flat curve in the diagram! A neat feature is that at at ~90% of the offsets, you still see 2 events cross in both figures '(a)' and '(b)', to indeed confirm that we just stretch the traces with no real further magic behind it...

So, the wavelet shape is constant in the vertical direction everywhere in figure '(a)', but is seriously stretched out in figure '(b)'.

From the page of the linked figure: "Note that stretching is confined mainly to large offsets and shallow times. For example, a waveform with a 30-Hz dominant frequency at 2000-m offset and t0 = 0.25 s shifts to nearly 10 Hz after NMO correction. Because of the stretched waveform at large offsets, stacking the NMO-corrected CMP gather will severely damage the shallow events. This problem can be circumvented by muting the stretched zones in the gather."

tl;dr: because the red and purple line in figure '(a)' must become the red and purple line in figure '(b)', the wavelets of particularly the purple line are stretched.

Answer 2

NMO stretch and associated mathematical relation i.e. $$ \frac{\Delta f}{f} = \frac{\Delta T_{NMO}}{T_0} $$ are precisely explained in the book- Seismic Data Analysis by Yilmaz. Mathematical part can be found in Appendix C2

The book is freely available.!

Answer 3

This is an important question - however, it has been asked and answered (with different content compared to above) here. Be that as it may, I think you should certainly compare what's been provided here to the link (and other resources too!).

I'll further supplement everything you can find with some insight that's helped me understand what happens with the apparent "stretch" when NMO-correction is applied to CDP(common depth point), CMP(common midpoint), and/or CIP(common image point) gathers (beyond the scope of this question).

When you see the classic equation and image referenced by @Eric, think to yourself: "What happens to the t term as x (geophones/hydrophones apart from and further away from the first sensor) increases?" You can play with this idea with different variables and constants, sample-by-sample.

Remember, that both the t₀ and v should remain constant for a correct stacking velocity for a single-impedance model (i.e. 2 layers). This becomes slightly more complicated with more layering, dipping horizons, and anisotropy. Don't let the latter part of this paragraph distract you from the main and simple idea you've posed though. The more complicated, real-world stuff can be handled later!