27
$\begingroup$

It has been observed that the shape of a meandering river is roughly circular, not sinusoidal (Leopold and Wolman 1960). It has also been observed that the following mathematical relationships tends to hold:.

$$\lambda \sim 11 w$$

and

$$r \sim 2.3w$$

Where $r$ is the radius of the circular bend, $w$ is the width of the river, and $\lambda$ is the length of the meander (wavelength), as labelled in the diagram below.

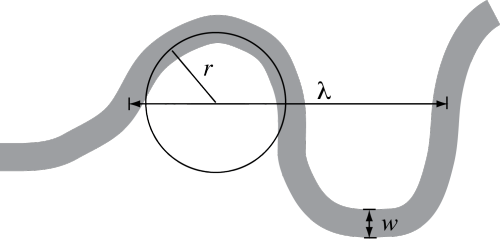
River equations diagram

Since this pattern was first observed in 1960, has a theoretical model of meandering rivers been obtained that explains the above observed relationships and if so, what is the theoretical explanation of this relationship?

Note: Given $r \sim 2.3 w$, it can be shown that $\lambda \sim 11 w$ from geometrical principles, or vice versa. For example $\lambda$ should be equal to

$$0.5w + 2r + w + 2r + 0.5w = 2w + 4r = 2w + 4(2.3w) = 11.2 w$$

So since the two equations are not completely independent, the question is how could either of these relations be determined from a theoretical basis.

References

Improve this question
$\endgroup$
10
  • $\begingroup$ I think this would be better for 1) mathematics stack exchange or 2) if we had mathjax. I would actually attempt the derivation if we had that capability but there is too much math without it. $\endgroup$
    – Neo
    Commented Apr 19, 2014 at 0:28
  • 8
    $\begingroup$ @Neo, Definitely not, it is not for maths.SE. I'm sure if I posted this to maths.SE they will say it is off-topic because it is not a mathematics problem, but a physics/geophysics problem. A mathematician cannot derive this because it is not a pure geometric problem, but a problem of the underlying model of meandering rivers and how they form. So unless the mathematician happens to be an expert in quantitative potamology, I don't think they will be able to solve it. It is for quantitative Earth scientists. $\endgroup$
    – Kenshin
    Commented Apr 19, 2014 at 0:31
  • $\begingroup$ I would really like to see your sources for those equations: I have found more general ones and they do not turn out, involve derivatives/integrals based on a variety of angles and meander amplitudes, as well as downstream length. Here are some book pages: books.google.com/… $\endgroup$
    – Neo
    Commented Apr 19, 2014 at 2:15
  • 2
    $\begingroup$ @Neo, I had a look at that book, and it includes my equation, R = 2.3W (page 182, last paragraph). The book has many other equations, but they are not in contradiction with the equations in my post, they are applications of the circular structure of the meandering. The paragraph gives reference to the Leopold and Wolman paper from 1960's and I believe the book is saying by plugging in appropriate constants into a more general formula, the r = 2.3w can be obtained. If you can find this out in more detail it would probably make a good answer. $\endgroup$
    – Kenshin
    Commented Apr 19, 2014 at 6:02
  • $\begingroup$ Ah you are correct; And while they do not include the spelled out derivation, they do include enough information I think that one could do it on their own. There are a few figures that the book references but google books does not show. perhaps ill work on it once mathjax is up, unless someone beats me to it. $\endgroup$
    – Neo
    Commented Apr 19, 2014 at 6:10

1 Answer 1

Reset to default
9
$\begingroup$

I'm gonna hazard a guess here: They can't. While I don't have any evidence for this claim, it seems likely that there are too many small-scale non-linear processes feeding in to the overall generation process to ever be able to sensibly derive any kind of analytical solution based on physical first-principles. I think that's what you're asking.

So, basically, these equations are an empirical model. The parameters don't have any relation to the physical processes that generated the meanders - they are just descriptions of the resultant shape. The generation process for a model like this is something along the lines of:

  1. Inspect the data qualitatively
  2. Come up with some potential models that could approximate what you're seeing. In this instance, sinusoidal and sequentially linked circles look like they might work.
  3. For each model, apply and verify:
    1. Attempt to fit the model to some of the data.
    2. Apply the model to some of the rest of the data, and check it's performance (also, pay attention to parsimony).
  4. Choose the model with the best performance as your model.
  5. With your model, perform some analysis of the model fit (residual analysis, etc). If it looks like there are patterns in the residuals that could be reasonably modelled, go back to 2. and come up with some model variants.

As far as I can tell from the referenced paper, this is roughly the process that the authors used. The values of each of the parameters are basically empirically obtained, to allow the model to best fit the majority of the data.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.