It is not an easy problem even for a simple object. The temperature will vary as the conditions change, but the usual approach to such problems is to find the equilibrium temperature for a set of conditions. This is, to find the final temperature that the object will reach if exposed to a given solar irradiation, with air at a given temperature, for given pressure, humidity, wind, etc.
Such equilibrium state will allow you to find the temperature by assuming energy balance. That can be stated as requiring that the incoming energy into the body equals the outgoing energy, or
$Ẹ_{in} = E_{out}$
To simplify the explanation let's assume the object was in equilibrium with air temperature during the night and we want to calculate its temperature onces the sun is shining on it. In this case the incoming energy will be function of the absorbed solar energy, therefore it will be function of the radiation intensity ($R_{sun}$) that depends on solar elevation, cloud cover, etc. (and can be obtain as a direct output of climate models), the albedo of the object ($\alpha$) and the area exposed to sunlight (i.e. coross-section) ($A$), that will be given by the shape of the object, solar elevation and azimuth. So, that is equivalent to:
$E_{in}=R_{sun} (1-\alpha) A$
Now, the energy leaving the object will have two components.
- Radiation: The object will cool down emitting electromagnetic radiation (mostly infrared) according to the Stefan-Boltzmann law.
- Conduction: As the object get hotter than the surrounding air, it will loose heat by thermal conduction into it.
So
$E_{out}=\kappa (T - T_{air}) + \varepsilon \sigma T^4 S$
Where $\kappa$ is the thermal conductivity of air, $T$ is the temperature of the object and $S$ its surface area. $\varepsilon$ is the emissivity (that is 1 for a black-body), and $\sigma$ the Stefan-Boltzmann constant.
The three equations above are sufficient to solve for the temperature of the object, but as you can see there are many parameters that depends on the object shape, and material, so it is impossible to generalize.
Also, in the absence of wind, the temperature of the air in contact with the object won't be the same as the surrounding air, further complicating the problem.
Furthermore, in addition to $A$, $\varepsilon$ and $\alpha$ can as well change with solar elevation and azimuth (if the object is made out different materials).
And to further complicate the problem, the thermal conductivity of the air $\kappa$ is a function of temperature, pressure and humidity. And it is not an easy relationship, it is usually tabulated or calculated using empirical formulas.
At engineeringtoolbox.com you can find tables and graphs of $\kappa$ for dry air, here is one example:
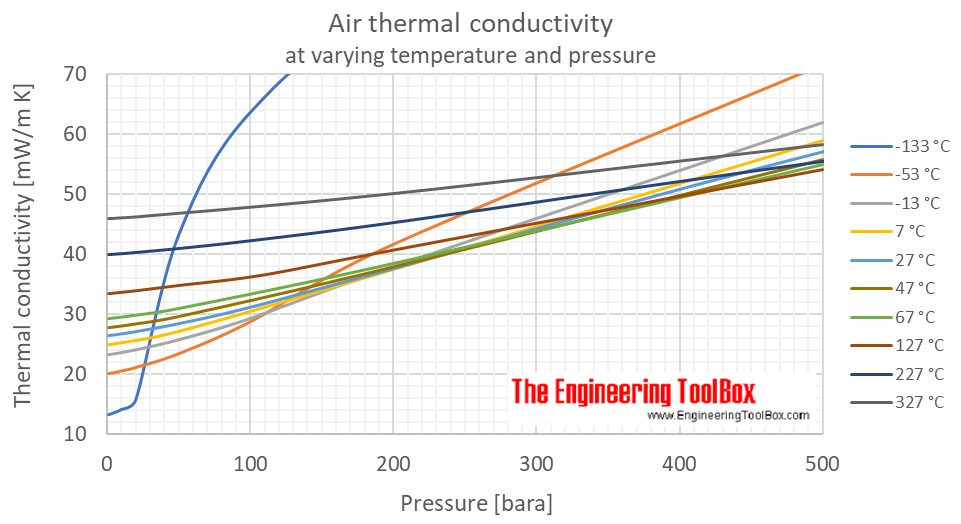
But the humidity have also an impact much more difficult to quantify, and whose importance grow with temperature. There is an article at electronics-cooling.com that explain the effect, summarized in the following graph:
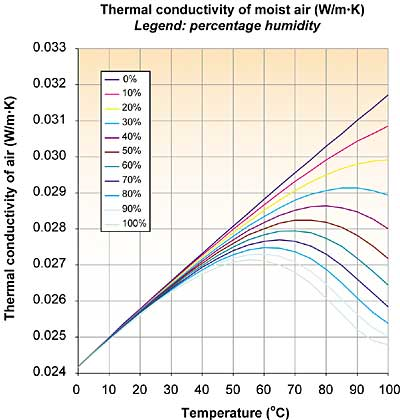
So, that is hard enough for an object, let alone for a person. In such case there would a lot more parameters that come to play. Like the amount of insulation provided by clothing, surface and moisture content of the skin exposed to the wind, body heat production, etc.
Apparent temperature
That been said, there are some attempts to estimate the perceived temperature for people including humidity, wind and solar radiation. The best example is perhaps the Wet-bulb globe temperature ($\mathbf{WBGT}$), that consist on the weighted mean of the readings of three different types of thermometers: A normal one, a wet-bulb one (affected by wind and humidity) and a very uncommon black-globe thermometer (affected by solar radiation).
The formula is:
$\mathbf{WBGT} = 0.7 \, T_{wet-bulb} + 0.2 \, T_{black-globe} + 0.1 \, T_{air}$
However, this value is not often used because is very location specific (depend on clouds for example). Nevertheless, perhaps a good approach you can take is to use actual solar irradiation from weather models to compute the temperature that a wet-bulb and a black-globe thermometer would produce, something that I think would be easy to achieve, and then compute the $\mathbf{WBGT}$ with the above formula.
Be aware that the $\mathbf{WBGT}$ value could be useful for people to know how cold/hot it will feel, but won't tell you anything about the actual temperature of different objects exposed to those conditions.

