Are any instruments today or at the time of the creation of the Panama canal capable of detecting a reduction in sea level from the creation of it? Given the size of the canal in comparison to the ocean I'm not sure that we have anything with the precision needed to measure this but I was curious if it was recorded or when the second and third lanes were added if it was recorded.
2
-
$\begingroup$ To put this in perspective, the Panama Canal contains somewhere around 0.1 cubic km of water. The Earth's oceans contain about 1.3 billion cubic km of water, with a surface area of 361 million square km. Filling the canal results in an average change of depth on the order of fractions of nanometers. $\endgroup$– Nuclear HoagieCommented Nov 16, 2020 at 19:48
-
$\begingroup$ @NuclearHoagie because of dam storage, it looks to be a bit larger in scale than 0.1 km^3, see my answer. But agree with the rough idea :-) $\endgroup$– JeopardyTempestCommented Nov 16, 2020 at 19:56
Add a comment
|
3 Answers
$\begingroup$
$\endgroup$
It's important to note that major man-made navigational canals often are often routed so that only a smaller bit of new waterways is dug; they are typically made by connecting existing waterways with smaller cut\cuts across higher mountainous areas, plus shortcuts in any place where straightening the route saves significantly.
It seems hard to find detailed construction maps (unfortunate!) But here is a map of the area before the canal was made from the USF historic maps archive:
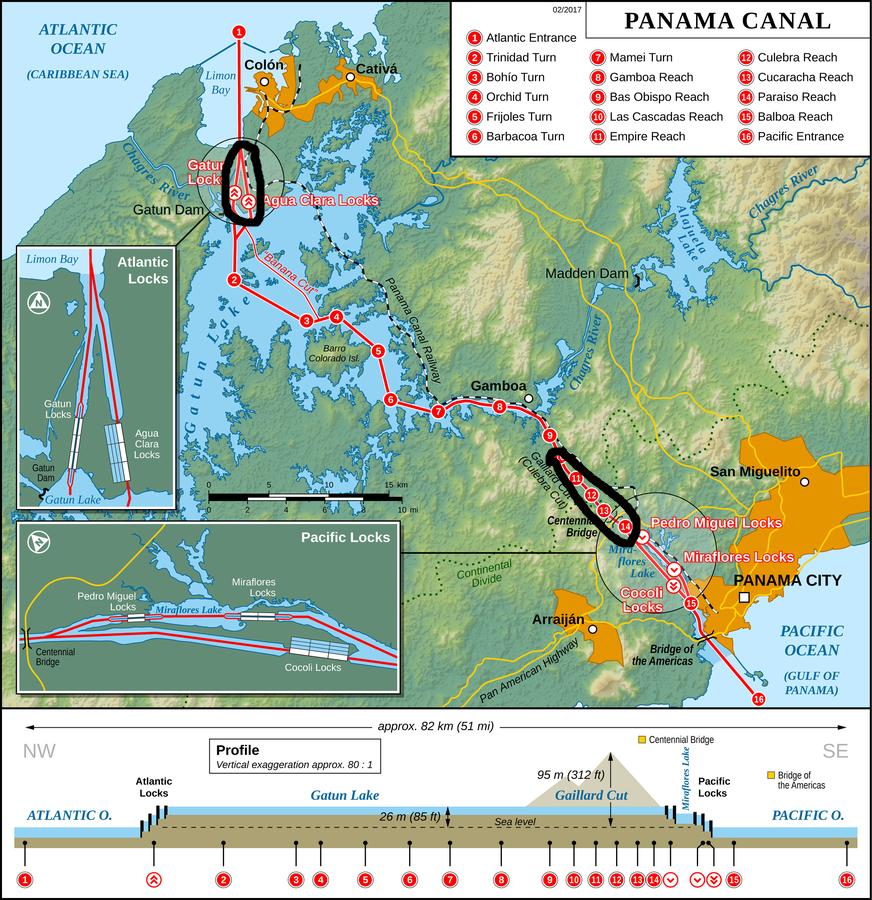
Comparing that to the Wikipedia map of the Canal shows two main areas of new waterway creation
However, the largest creation on the map appears to be the expanse of Gatun Lake created by damming the river to create a wider channel (with other connected benefits like power and water storage). Damming rivers is of course a widespread practice around the world for purposes including water regulation, power generation and agriculture.
Looking at rough estimates of water changes (hopefully with no errors!):
Note these use the US\UK definitions of billion as $10^9$ and trillion as $10^{12}$. Replace billion with millard and trillion with billion in other regions.
- The Culebra Cut "has an average depth of about 43 feet (13 metres) and extends some 8 miles (13 km) to the Pedro Miguel Locks" and "throughout its length the canal has a minimum bottom width of 500 feet (150 metres)" [Brittanica].
So that would be about 7 billion gallons (26 billion liters). - The Wikipedia articles lists Gatun Lake as 4.2 million acre*feet.
That's 1.4 trillion gallons (5.2 trillion liters).
Lake Alajuela is another 150 billion gallons (600 billion liters).
Given that the lakes are the significant amounts of water, 2 trillion gallons (8 trillion liters) would seem an extremely generous estimate for the amount of additional water impounded by the entire system (including widening the river and the water in the Gatun, Pedro Migual, and Miraflores Locks). If assumed all of that ends up being removed from the ocean, averaged over the 140 million square miles (362 million square km) of ocean surface area, that would be roughly 20 micrometers of depth (less than 1/1000th of an inch), comparable to the width of a human hair. Again, remember that's a very generous estimate.
Given that Wikipedia lists 48 reservoirs around the world that each are more than twice the amount of the water estimated above, it would seem more significant to ask how much those remove in total from ocean. Still, all those combined would seem only to be on the order of perhaps centimeters of depth lost in aggregate.
answered Nov 16, 2020 at 19:11
$\begingroup$
$\endgroup$
1
Another way to look at this is to consider that everything that was dug out was then filled by sea water. Based on this (non realistic, see below) hypothesis, we need the amount of excavated rocks as a proxy for water volume. The Panama Canal Museum "interesting facts" webpage has:
- Excavation by French useful to present Canal (cubic yards) 29,908,000
- Amount of excavation by Americans in cubic yards 232,353,000
Yielding a total of 262 261 000 cubic yards, or $\sim$0.2 km$^3$ of excavated material. Now if you take this volume of water from the 362 million square kilometers area of the World Ocean, you get a depth of:
$\frac{0.2}{362000000} = 5.5 \times 10^{-10}$ km, or 0.55 microns.
However, the premise is false because most of the canal is filled with fresh water, not sea water. The Gatún Lake mentioned in @JeopardyTempest's answer is fed by a river.
answered Nov 17, 2020 at 9:57
-
1$\begingroup$ This was my first thought of the way to do the math too :-) ... but I came to think about the potential that probably not nearly everything dug out was filled with water, because digging through the mountains likely required a significant amount of created slope that wasn't to be filled with water. And yeah, the damming filled in areas that were never dug out. But still glad to see it done +1 $\endgroup$ Commented Nov 18, 2020 at 3:55
$\begingroup$
$\endgroup$
1
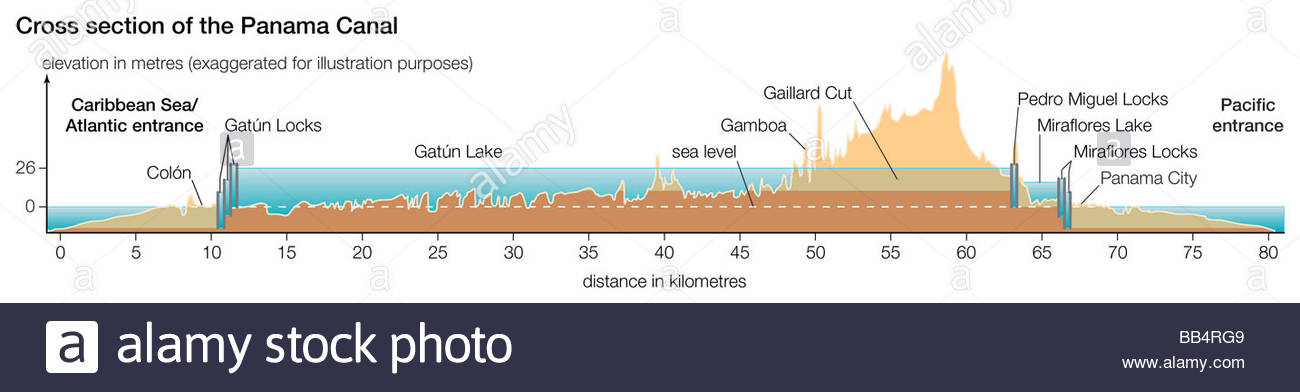
Since the Panama Canal isn't directly connected to the ocean and the water is overwhelmingly above the ocean sea surface, there was no reduction in sea level. See diagram:
-
2$\begingroup$ I don't see why the fact that the canal is above sea level, or the fact that it's not directly connected to the ocean would make any difference for this question - that water had to come from somewhere. One could empty the ocean by pumping it into a sufficiently large above-ground tank. Whether we can measure the drop in sea level or not, you can't fill the Panama Canal "for free" without taking that water from somewhere else, regardless of the canal's height or connection to the ocean. $\endgroup$ Commented Nov 16, 2020 at 20:45