2
$\begingroup$

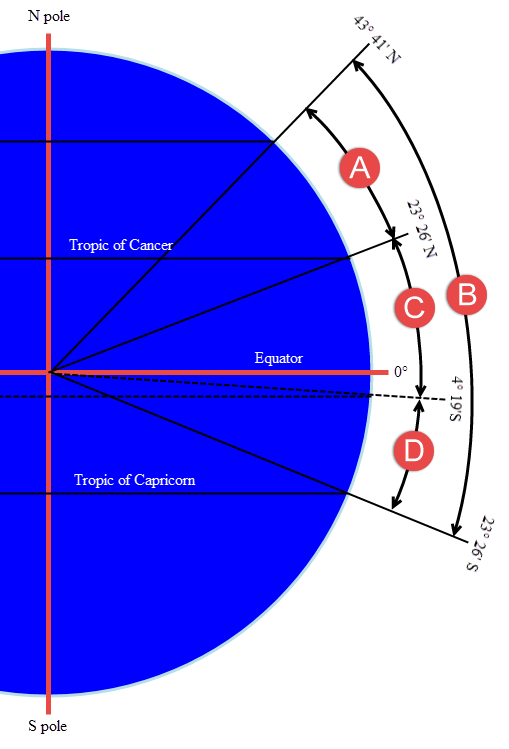
I am given examples of this for Brampton (Canada) (43° 41’ latitude)

On the Summer Solstice you do latitude minus the Earth’s axis tilt which is 23° 26’, so 43° 41’ - 23° 26’ = 20° 15’.

On the Winter Solstice you do latitude plus the Earth's axial tilt, so 43.° 41’ + 23° 26’ = 67° 07’.

I found that the latitude of Kinshasa is approximately 4° 19'S but I'm not sure how we would use this information to solve the question. If we would subtract from the Earth axis tilt we would get a negative value, which doesn't make sense to me. From what I know is that the Summer Solstice occurs when the sun is directly over the Tropic of Cancer, which is located at 23.5° latitude. The Winter Solstice occurs when the sun is directly over the Tropic of Capricorn, which is located at 23.5° south of the equator. Kinshasa is located in the tropic of Capricorn. So can you help me understand how to calculate these?

Improve this question
$\endgroup$
2
  • $\begingroup$ Some things to bear in mind. In the northern hemisphere, for all latitudes north of the Tropic of Cancer, such as Canada & Europe, the sun will always appear in the southern part of the sky. Similarly in the southern hemisphere, for all latitudes south of the Tropic of Capricorn the sun will always appear in the northern part of the sky. For both tropical zones, Cancer & Capricorn, depending on the time of year, the sun will be in the southern part of the sky, directly above or to the north. ... $\endgroup$
    – Fred
    Commented Mar 5, 2022 at 22:31
  • $\begingroup$ ... Also, when the northern hemisphere has its summer solstice the southern hemisphere has its winter solstice. While Canadians & Europeans might be dreaming of a white Christmas, Australians are looking forward to spending Christmas on the beach keeping cool. $\endgroup$
    – Fred
    Commented Mar 5, 2022 at 22:34

1 Answer 1

Reset to default
2
$\begingroup$

A simple diagram may help to explain the calculations. 23° 26' is the north and south latitudes where the Sun is overhead on the solstices. The angles that you give for Brampton Canada are the zenith distance of the Sun; that is, the angle between the point overhead (the zenith) and the Sun at noon. sun's position

  • For summer at Brampton, A = 43° 41' - 23° 26' = 20° 15'.
  • For winter at Brampton, B = 43° 41' + 23° 26' = 67° 07'. (The Sun is further from the zenith and lower in the sky in winter.)
  • For summer at Kinshasa, D = 23° 26' - 4° 19' = 19° 07'.
  • For winter at Kinshasa, C = 23° 26' + 4° 19' = 27° 45'. (The Sun is further from the zenith and lower in the sky in winter.)
Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.