Why isn't the side of the earth facing the sun completely illuminated by sunlight from the north to the south pole every day of the year?

Question

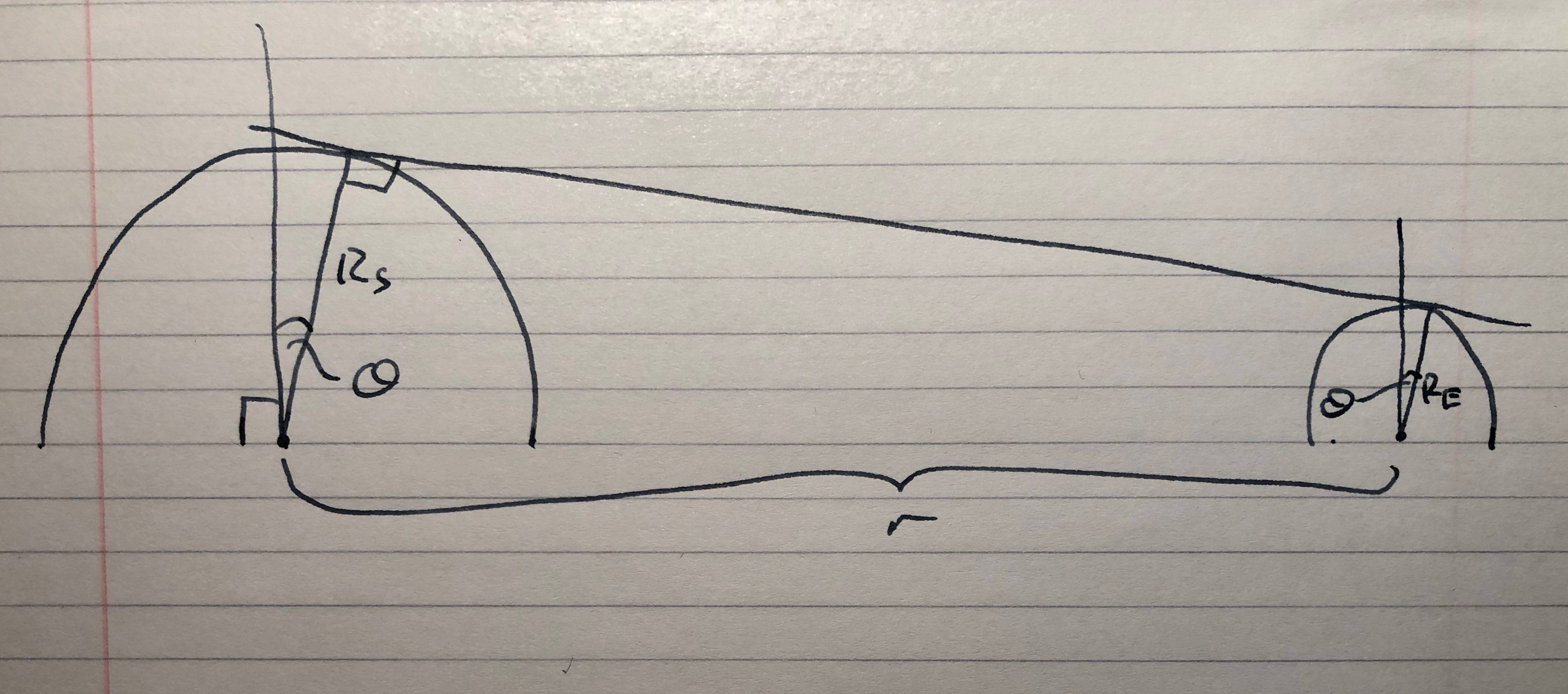
We all know that the earth rotates every 24 hours, that it is tilted 23.5 degrees, that it circles the sun every 365 days, and that it is located approximately 148,600,000 km (92,333,000 miles) from the Sun.

We also know that we have 5 major circles of latitude, from north to South: the Arctic circle at 66.5 degrees north, the Tropic of Cancer at 23.5 north; the Equator at 0: the Tropic of Capricorn at 23.5 south, and the Antarctic Circle at 66.5 south.

I have read previous answers regarding questions similar to mine but feel that the question(s) were perhaps misinterpreted as to meaning why isn't Earths side opposite the sun illuminated given the sun's size, so I want to be more specific.

Why isn't the side of the earth facing the sun completely illuminated by sunlight from the north to the south pole every day of the year?

Because if the The Earths diameter is 12,740 km (7,917 miles) and the Sun's is 1,392,700 km (865,370 miles), or about 109 times greater, it would seem logical that the sun's rays would provide sunlight on the face of the Earth pointed towards the Sun, completely from the North Pole to the South Pole, and yet we know that it doesn't... Why so?

Answer 1

If the diameter of the Sun is $R_S$, the diameter of the Earth is $R_E$, and the orbital radius of the Earth is $r$, as in the drawing below (sorry about the quality), then you can do some geometry and compute $\theta$, which is the angle at which the light from the edge of the Sun's disk as seen from Earth is tangent to the Earth's surface referenced to a line between the centres of the Sun and the Earth.

The expression for $\theta$ comes out to be

$$\theta = \sin^{-1}\left(\frac{R_S - R_E}{r}\right)$$

(You get this by extending the tangent line and the line connecting the centres, realising that this makes two similar right triangles with the angle at the tip being $\theta$, and the base of the larger one being $R_S$, then working out what the hypotenuse of the larger triangle is, which gives you $\sin\theta$.)

Since $\theta$ is small we can just say $\sin \theta = \theta$ in the usual way, and plugging in values we get $\theta \approx 0.0046\,\mathrm{rad}$.

If the angle at which the Earth's rotation axis is tilted is more than $\theta$, then there will be a period of the year during which the poles are not illuminated as the point at which the Sun's light is tangent to the Earth's surface is sunward of the pole. Well, the angle of tilt is $23.5^\circ \approx 0.41\,\mathrm{rad}$, which is about $90\theta$.

Answer 2

You give the answer in your question when you say the earth is tilted (on its axis). Because of this tilt, in winter the northern hemisphere is tilted away from the sun, so for a short while the north pole experiences complete darkness, while at the south pole the sun is in the sky for 24 hours a day and it is summer.

In the southern winter you have the same situation in reverse, and it is the north pole on which the sun never sets. That's why it's summer in the north. Because of the Earth's tilt, the south pole is pointing away from the sun at that time of year. That is why you can't have sunshine from pole to pole every day of the year.

I don't see what the sun's diameter has got to do with it. As seen from Earth, its diameter is tiny, regardless of the fact that it is over 860,000 miles across.

Answer 3

The sun does actually illuminate the earth with a disk a bit larger than if it would be a point light source.

But that extra Halo which is generated by say a ray coming off the most easterly point on the sun's apparent disk to the most westerly point on the earth lit disk and same for other points on the perimeter of the sun hitting the surface of the earth diagonally is very small, it is half of the sun's apparent angle which is near 32" arcseconds.

If sun were big enough to lite up the entire face of the Earth from North Pole to South Pole it needed to be as big as to occupy a vast area in the sky as big as almost a quarter of the visible sky. 23+23=46 degrees apparent angle of view.

Answer 4

Michael Walsby's answer is correct, but I thought I'd try putting it another way:

The sun does illuminate the side of the earth that faces it, not just every day of the year but every moment of the year.[1] But, because of the tilt that you have mentioned, the "side" facing the sun does not usually correspond to a hemisphere that starts and ends at the north and south poles.

[1] except during eclipses