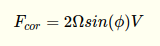I came to know that Coriolis effect is maximum at the poles and negligible at the equator. I could not figure a logical meaning behind it. Could someone please explain? Also, does it have anything to do with the trade winds and the ITCZ?
-
4$\begingroup$ @SheelaRampart The Coriolis force is not exactly zero at the equator. The horizontal component is zero but the vertical component is non zero at the equator. $\endgroup$– user1066Commented Aug 17, 2022 at 10:28
-
1$\begingroup$ @SheilaRampart courses.eas.ualberta.ca/eas570/coriolis_force.pdf $\endgroup$– user1066Commented Aug 17, 2022 at 10:34
-
3$\begingroup$ Could you re-phrase "I came to know that Coriolis effect is maximum at the poles and negligible at the equator…"? How did you come to know that, and what did it mean to you? I ask because without those answers, it might seem obvious there was no logical meaning, solely because you did not at all "come to know" anything. Rather, you were told it was so, which is not the same thing by any manner of means. In your case, did you really "come to know" or did you merely accept what you were told? $\endgroup$– Robbie GoodwinCommented Aug 17, 2022 at 21:35
-
1$\begingroup$ A great question... please don't be unduly unsettled by Robbie's semantics, if you tell us more about how well you understand Coriolis it can help certainly, but I think most of us recognize that knowing isn't necessarily an intimate familiarity with all facets of something (the definition of the word "know" includes things like "to be cognizant or aware, as of some fact". In the education world, there's a million things you are to know for a test... the greater hopes is that you'll understand, which asking questions like this are a great way to do :-) ) $\endgroup$– JeopardyTempestCommented Aug 18, 2022 at 4:41
-
1$\begingroup$ Given that the Coriolis effect makes weather systems rotate counterclockwise in the Northern hemisphere and clockwise in the Southern hemisphere, in which direction would the horizontal component of the Coriolis force act at the equator if it were to exist? $\endgroup$– ChristianCommented Aug 18, 2022 at 23:49
3 Answers
The Coriolis effect typically refers to the horizontal component of the fictitious acceleration that appears in an Earth-centered, Earth-fixed frame of reference (and also in any other Earth-fixed frame of reference such as local east-north-up) due to a horizontal velocity.
A more general expression is that the acceleration due to the Coriolis effect is $\boldsymbol{\mathrm a}_c = -2\boldsymbol\Omega\times\boldsymbol {\mathrm v}$, where $\boldsymbol{\mathrm v}$ is the velocity vector with respect to the rotating Earth, $\boldsymbol\Omega$ is the Earth's angular velocity vector with respect to inertial, and $\boldsymbol{\mathrm a}_c$ is the resulting Coriolis acceleration.
In local east-north-up coordinates, the Earth's angular velocity vector is $\boldsymbol\Omega = \omega\,(0, \cos\phi, \sin\phi)$, where $\phi$ is the geocentric (not geodetic) latitude and $\omega$ is the Earth's sidereal rotation rate. At the equator, $\phi=0$, so $\boldsymbol\Omega = (0,\omega,0)$ here. The Coriolis acceleration $-2\boldsymbol\Omega\times\boldsymbol {\mathrm v}$ has zero horizontal components at the equator if the velocity vector is purely horizontal.
However, there is a non-zero vertical component to the Coriolis effect. If you drop a ball from the top of a tall vertical tower at the equator the ball will land to the a bit to the east of the base of the tower due to the Coriolis effect. On the other hand, if you shoot a ball straight up at the equator, the ball will land a bit to the west of where you shot it from.
You tagged this question meteorology. Winds are mostly horizontal. The horizontal component of the Coriolis effect due to a horizontal wind at the equator is zero.
David's answer is comprehensive, but perhaps a little technical for the level of the question, so I'll try to offer another angle.
Coriolis is an apparent force that causes an air parcel to curve to the right in the northern hemisphere and to the left in the SH and can be understood by a conservation of angular momentum. With regards to how Earth's rotation affects the apparent horizontal movement of wind, these figure gives a nice summary:
We can see that closer to the poles, an aircraft will appear to have more Earth "move" beneath them while in the air. Another way to visualize the effects of Earth's rotation is imagining a person standing at the equator and at the pole. Equator-person will feel no rotation along their vertical axis with respect to the planet, while pole-person will continuously spin in place with respect to the planet's axis.
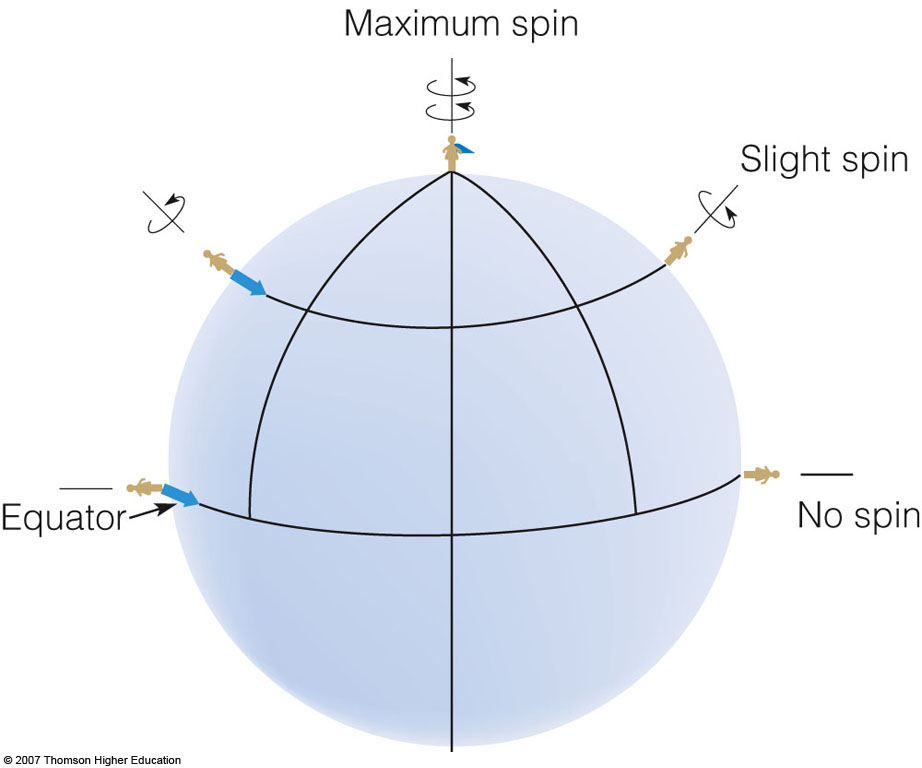 Because air parcels are in constant motion over a rotating surface, the amount of apparent horizontal deflection is determined by the latitude of the air.
Because air parcels are in constant motion over a rotating surface, the amount of apparent horizontal deflection is determined by the latitude of the air.
A simplified version of north/south Coriolis force operating on horizontal winds is given by:
Where omega (Ω) is the rate of earth's rotation, or angular velocity, (7.292 x 10-5 sec-1), and phi (ϕ) is the latitude of the wind velocity component (V) in question. Because Earth spins counter-clockwise, the apparent deflection of wind is 90° to the right in the northern hemisphere and 90° to the left in the SH.
From the equation we can see that if the latitude is zero, then the horizontal north-south deflection contribution of Coriolis is zero. It's important to note that Coriolis has more components than north-south contributions, like in the vertical and east-west, but for the purposes of wrapping your head around the basics this should suffice.
-
2$\begingroup$ Perhaps useful to mentally compare the globe diagram to a cylinder. On a cylinder, moving along it never takes you closer or farther from the axis of rotation. Near the equator is like this. Or to put it another way, a cylinder is all equator, no non-zero latitudes. (Ignoring the flat discs at the ends of a cylinder; I'm just talking about the curved surface.) $\endgroup$ Commented Aug 19, 2022 at 20:16
-
$\begingroup$ @PeterCordes love that way of thinking! $\endgroup$– BbJugCommented Aug 20, 2022 at 13:21
-
1$\begingroup$ Nice explanation. Here is another that goes into more about why we call this apparent motion a force. Coriolis Force: Direction Perpendicular to Rotation Axis Visualization $\endgroup$ Commented Jan 7, 2023 at 18:54
The Coriolis effect is an effect that applies in a rotating frame of reference when one changes one's distance from the axis of rotation. (Going towards the axis of rotation means that a constant linear velocity would result in an increase in angular velocity, causing an apparent force in the direction of rotation, and vice versa for going away from the axis of rotation). At the equator, the axis of rotation of the Earth is parallel to the tangent plane of the Earth, so traveling on the Earth's surface does not change the distance to the axis. Vertical motion does change the distance, so there is a Coriolis effect on falling objects.
There are also symmetry arguments showing that the Coriolis effect at the equator is zero. At the equator, there is nothing distinguishing North from South, so the effect of moving in either direction should be the same, so it must be zero. When you get away from the equator, one direction is moving towards the closest pole, and the other is moving away, and that breaks the symmetry. There's also the argument that since the effect is opposite in the two hemispheres, it must go to zero somewhere, and the most obvious place for that is the equator.