What are the criteria to determine if river A is a major or minor tributary to another River B?
Is stream order the only deciding factor i.e. the highest order is the main stem, one less order is a major tributary and one more lesser order is a minor tributary?
I haven't been able to find any literature on this online.
This is a question of topology. There are three major attempts to order streams from small to larger.
The first was established by Horton (1941) who established the concept of drainage composition. To establish the relative importance of streams in a network Horton suggested to investigate each junction and to set the stream that entered the junction at the highest angle as a stream of lower importance. By starting at the mouth of a river, one can thus establish which would be the main trunk of a river and which are tributaries of smaller and smaller significance. The order number of streams cannot be determined until the entire tree of streams have been ordered. In the figure on Strahler stream order the diagonal would be the main trunk of the river and have order three. The only order two trunk would be the one labelled two turning down from three in the figure.
Strahler, 1952 took the concept further and established the concept of stream order. In this approach the basic stream is the smallest source tributaries. When two streams of order one meet they form a stream of order two. It takes two of the same order to make a stream of a larger order so a stream of order two and one will not increase the order number, the result is still two. The following image provides an example of Strahler's stream order:
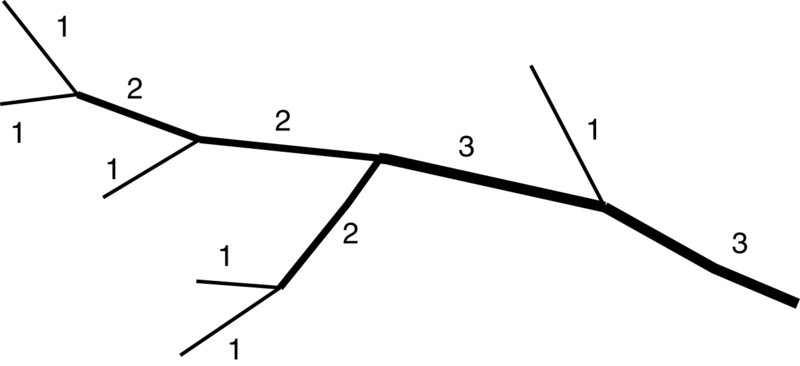
Strahler stream order concept. Image from Wikipedia commons
The stream order allows one to calculate an assortment of statistical measures that characterizes a drainage basin and it is possible to use these characteristics in, for example, flood prediction.
Shreve (1967) took the ideas of Horton and Strahler further by introducing magnitude, now called Shreve Magnitude. This concept differs from Strahler's in that all streams are additive so that as soon as one stream is added to another the resulting stream is the sum of the two tributaries. The result is that the system can be seen as reflecting discharge, assuming all first order streams are of similar size. In the figure above the largest magnitude would be six, this is also the sum of all order one streams.
So establishing the main trunk of a river is not necessarily simple. Hortons method does this but is at the same time relying on geometry and rivers may be heavily influenced by geology to generate odd river patterns.
References:
Horton, R.E., 1945. Erosional development of streams and their drainage basins: Hydrophysical approach to quantitative morphology. Geological Society of America Bulletin, 56, 275-370. doi: 10.1130/0016-7606(1945)56[275:EDOSAT]2.0.CO;2
Shreve, R.L., 1967. Infinite topologically random channel networks. Journal of Geology 75, 178–186.
Strahler, A.N., 1952. Hypsometric (area-altitude) analysis of erosional topology. Geological Society of America Bulletin, 63 (11), 1117–1142, doi:10.1130/0016-7606(1952)63[1117:HAAOET]2.0.CO;2.
