I don't see this point made, so I'll just add it. In very high gravitation situations orbital energy can be lost to "relativity" or more specifically, gravity waves. The primary relativistic effect that the Sun's gravity has on Mercury is an increase in it's precession, as @CamiloRada pointed out, and see more details here.
A secondary relativistic effect is orbital decay, or spiraling in. This is observable in tight orbits around neutron stars or black holes. The effect around a regular star would be tiny but non zero.
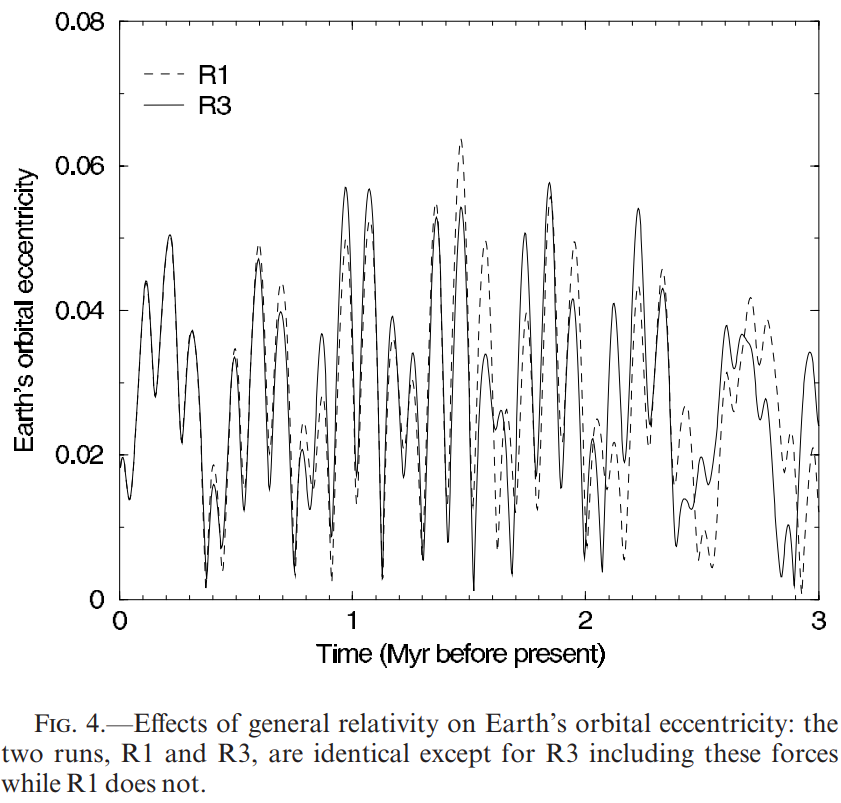
This article gives some numbers for this effect on the Earth.
Gravitational radiation is incredibly subtle and weak in its effects.
The Earth, as it orbits the Sun, loses energy due to this radiation
and spirals inwards. But it will take 10^150 years for this process to
occur.
That's a preposterously long period of time. Mercury would certainly be effected significantly more than Earth, (I can't do the math, maybe someone here will step up), but even at mercury distance the energy loss to gravity waves and spiraling-in would be slow to the point of irrelevance compared to other effects.
Our sun, for example, grows larger and brighter over it's lifetime. Planets atmosphere's change, either stripped off or in the case of Earth, affected by photosynthesis and other processes, and the Sun loses mass over it's lifetime, which causes the planets, on average to move outwards, though inward migration is also thought to be possible and the planets can affect each other's orbits. These changes to climate are all likely to be significantly greater than relativistic spiraling-in.
For maximum "spiral-in" effect, you'd probably want a small star and a planet with a very tight orbit of just days, but even that would likely be too small to have significant effects and a planet that orbited faster than it's star rotates would likely experience some tidal forces drawing it inwards. The tidal drawing-in would likely be far greater. It's hard to imagine a situation where the relativistic spiraling-in would have much effect between a planet and star.
Now a planet in a super-tight orbit around a white-dwarf star . . . maybe, but the planet would likely get torn apart inside the Roche limit long before you got it close enough for any measurably interesting relativistic spiraling-in.
A word on precession, precession affects the Earth's climate because the Earth has a land dominant hemisphere and a water dominant hemisphere. Precession also works with the seasons leading to warmer or colder winters or summers. If there's low axial tilt, then precession won't have much effect on a planet's climate. Mercury has minimal axial tilt and no significant variation between it's two hemispheres, so it's unlikely that Mercury's precession changes it's climate much. Certainly not like the effect it has on Earth, such as turning ice ages on or off.
Precession is also mostly Newtonian. Relativity just adds a percentage point or two to the rate of precession in the case of Mercury.