The perigean spring tides arise when the spring tide occurs during the time of shortest distance between Moon and Earth. As the full moon on October 15-16, 2016 occurred near perigee, then it can be consider a "supermoon" even if the difference in Moon size was not that great. The fact is that the October full moon was not even the largest of the year; that will occur in November:
The November 14, 2016 full moon – closest and largest full moon is 2016 – will also be the largest full moon thus far in the 21st century (2001 to 2100). It’ll be the closest encounter between the Earth and moon until November 25, 2034.
Thus, the mid-October was exceptional in a sense, but will be surpassed by the November event.
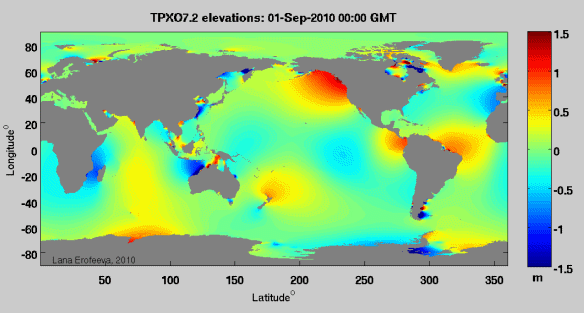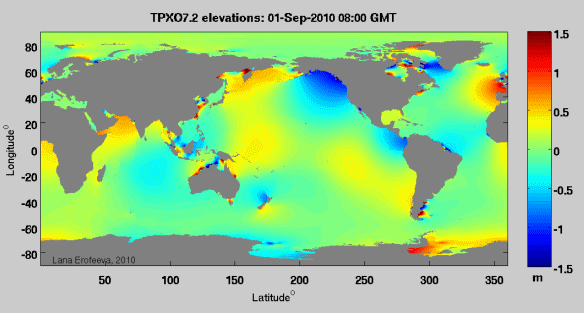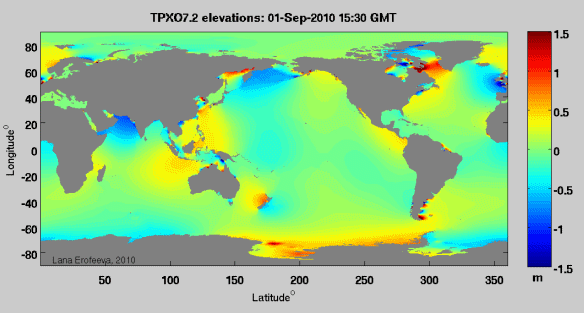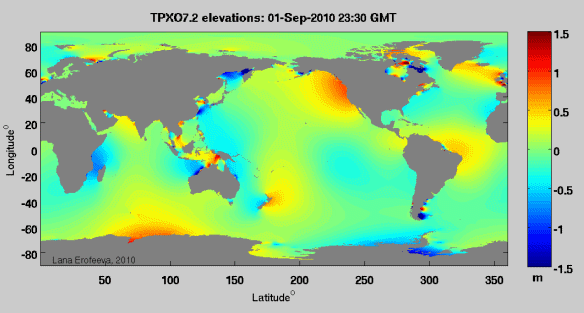
With regard to the effect on tides, the combination of the $M_2$ and $S_2$ tidal constituents gives rise to the spring/neap cycle. Meanwhile, the $N_2$ tidal constituent arises from the fluctuating distance of the Moon from Earth and its frequency is 1 cycle/month less than that of $M_2$ and 3 cycles/month less than that of $S_2$.
We can resynthesize a time series of tidal elevation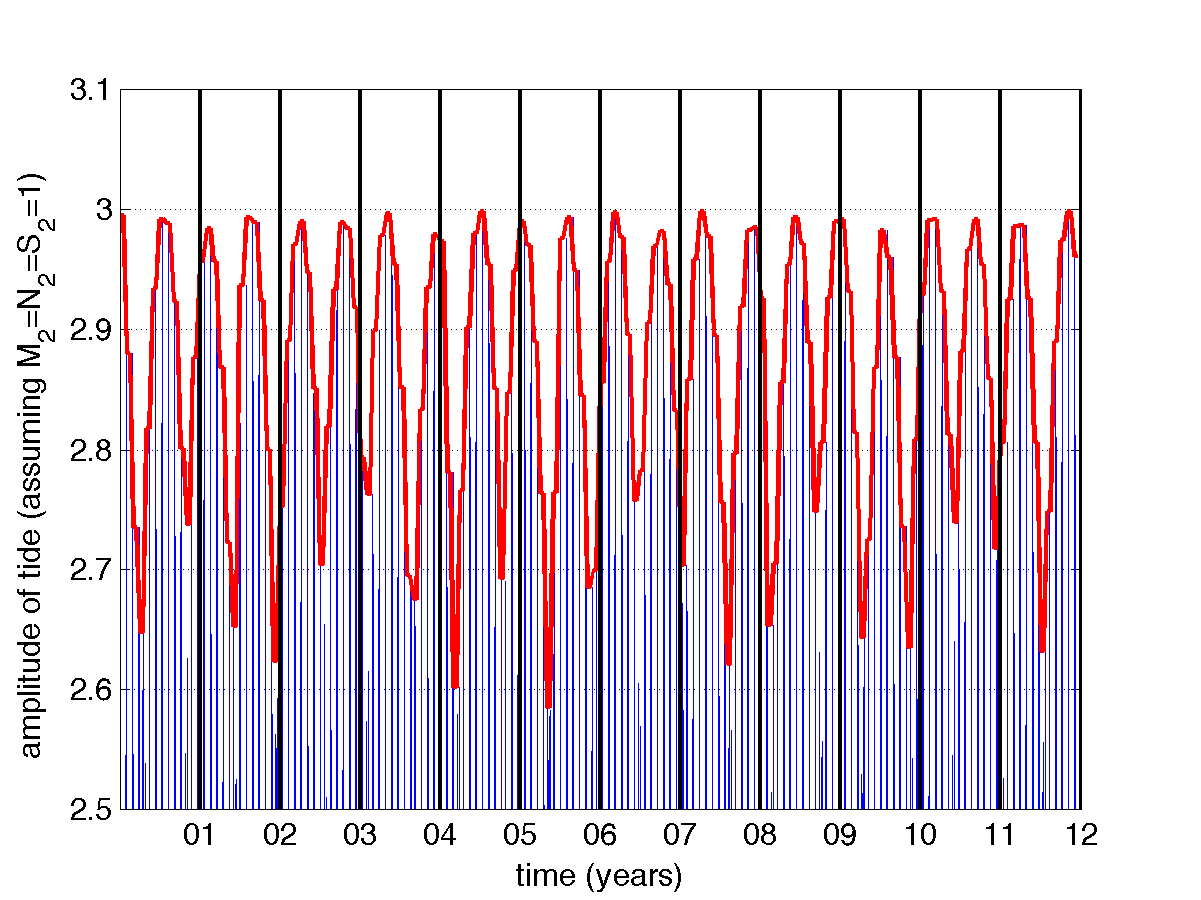 
based on only these three tidal constituents ($M_2$, $S_2$, and $N_2$). The resulting time series of amplitudes has an envelop that shows the size of the spring tides. As can be seen in the figure, even including only these three constituents, the resulting variability in the size of the spring tides (12 years shown) is pretty high. The problem is how to define "king tides". There are some spring tides that are larger than others, but even among the largest there is variability. This is where the uncertainty on the definition of the recurrence interval of the "king tides" comes from. Ultimately, it seems that having ~2 peaks ("king tides") per year is a good approximation. Including 3 constituents is a significant simplification and the entire series gets more complicated when additional constituents are included and defining a "king tide" is also more difficult.
. 