5
$\begingroup$

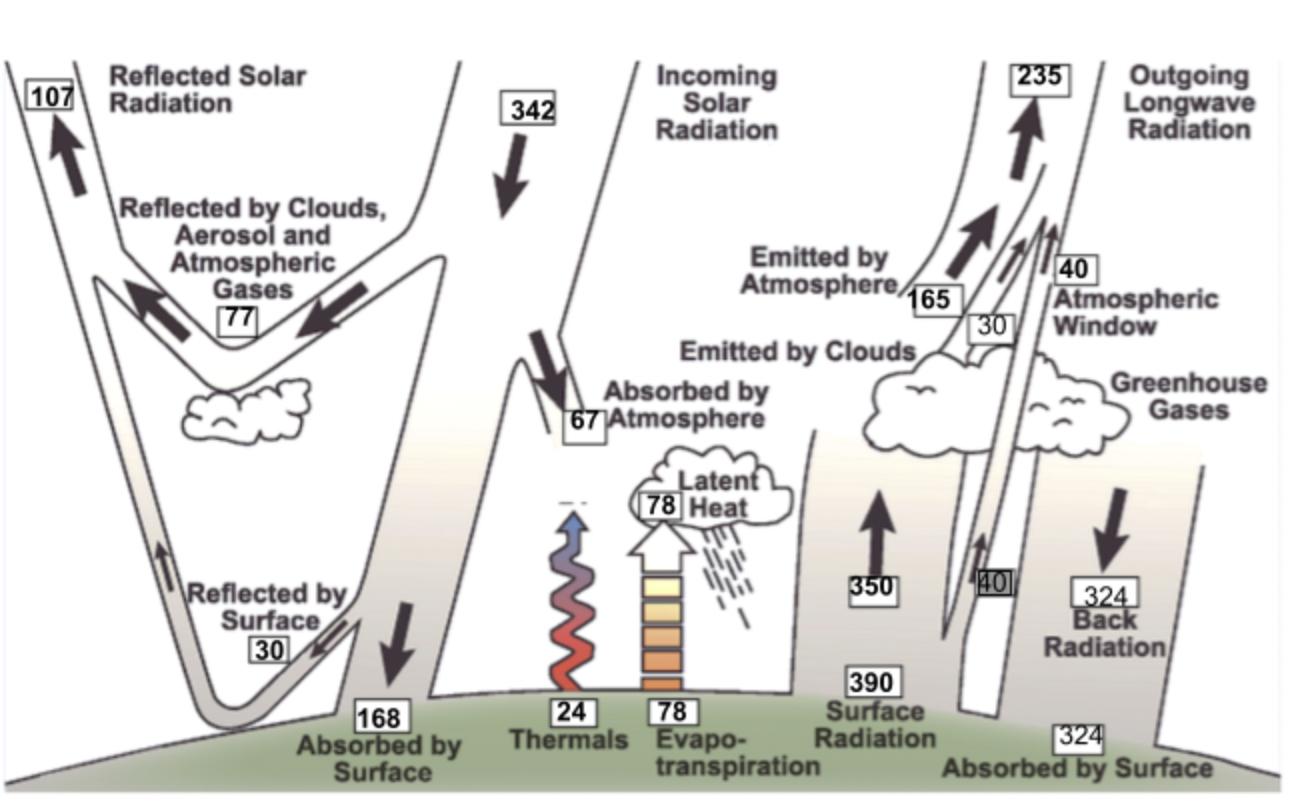
enter image description here

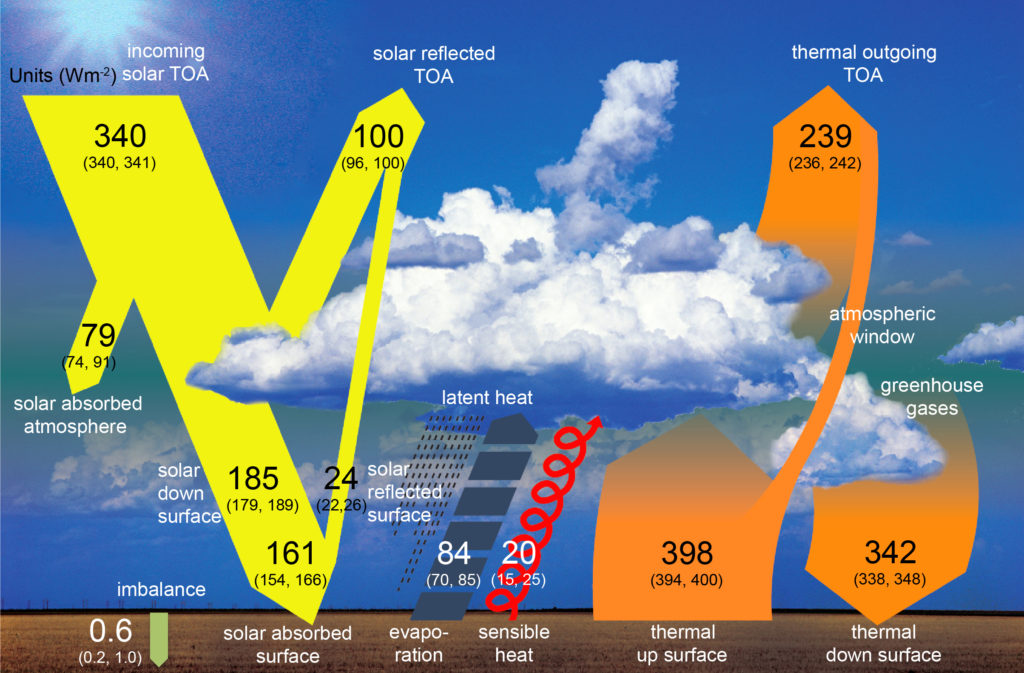
How would one go about calculating the value for back radiation(324 in this diagram) if it wasn't provided.

According to this website, it is "equivalent to 100 percent of the incoming solar energy" but from this diagram, that statement appears to be false as the incoming solar energy is 342 while the back radiation is 324.

Could someone tell me how to do it? Or is it just not possible?

Improve this question
$\endgroup$
6
  • $\begingroup$ Incoming solar energy absorbed by the planet is 235 watts per square meter per the diagram in the question (and that diagram is dated; it was produced in 1997). That value (235) is less that 324. $\endgroup$
    – David Hammen
    Commented Sep 5, 2021 at 14:34
  • $\begingroup$ So are you saying the diagram is wrong or something? Also, what does the incoming solar energy absorbed tell us about the back radiation? Thanks $\endgroup$
    – import_hill
    Commented Sep 5, 2021 at 14:38
  • $\begingroup$ The diagram clearly shows that only 235 watts per square meter of the incoming solar energy is absorbed by the Earth. The rest (107 watts per square meter) is reflected by clouds, the Earth's surface, etc. $\endgroup$
    – David Hammen
    Commented Sep 5, 2021 at 14:40
  • 1
    $\begingroup$ How would we use that to calculate for the back radiation? I've never done this before. $\endgroup$
    – import_hill
    Commented Sep 5, 2021 at 14:42
  • 1
    $\begingroup$ I presume you'd need the full solution for the steady-state of the radiation field, i.e. solve the two-stream radiative transport equations for that. Once you have the full solution, you can substract the diffusive radiation from your first atmospheric layer to get the backradiation. I can point you to a source for the full solution for irradiated exoplanets, i'm not familiar with the terrestrial literature. $\endgroup$
    – AtmosphericPrisonEscape
    Commented Sep 9, 2021 at 13:44

1 Answer 1

Reset to default
1
$\begingroup$

The back radiation looks to be calculated by summing all radiation components that the atmosphere (including clouds) absorbs and then subtracting the radiation it emits upwards. What remains is the radiation it emits downwards (i.e. back radiation). In the figure it is indeed: $$ (67\ \mathrm{Wm^{-2}} + 24\ \mathrm{Wm^{-2}} + 78\ \mathrm{Wm^{-2}} + 350\ \mathrm{Wm^{-2}}) - (165\ \mathrm{Wm^{-2}} + 30\ \mathrm{Wm^{-2}}) = 324\ \mathrm{Wm^{-2}}$$

The "equivalent to 100 percent of the incoming solar energy" reference in NASA's website is a rough approximation and it is not a result of some conservation law that says the two amounts must be exactly equal. It only means "we have estimated that the atmosphere's back radiation is approximately equal to the incoming solar radiation" (and indeed $324\ \mathrm{Wm^{-2}}$ is approximately 100 percent of $342\ \mathrm{Wm^{-2}}$). Other similar estimations are suggesting slightly different amounts and percentages, but all are more or less quite close to each other for each radiation component. See for example the respective figure in Chapter 2 of 2013 IPCC Report:

IPCC 2013, The Physical Science Basis, Chapter 2, Figure 2.11

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.