What place on Earth is closest to the Sun?

Question

What is the location on Earth that is closest to the Sun?

I've seen this question asked many times, and answered in varied and contradictory ways:

• The most common answer is “the summit of Chimborazo volcano in Ecuador”. This volcano is the point on Earth's surface that is furthest from the center of Earth, and that is then equated to being the closest to the Sun. This is very commonly spoken of around the Chimborazo volcano area and among the people involved with tourism there (here is an example of this answer)

• Others argue that it is Cayambe volcano in Ecuador, it being the highest point along the equatorial line (answer example).

• Others say Mount Everest in Nepal/China because it is the highest point on Earth (example).

• And others argue it is Sairecabur volcano in Chile/Bolivia, because it is the highest point at the latitude which is closest to the Sun on January 5th, when the perihelion happens (i.e. the point in Earth's orbit that is closest to the sun) (example).

• A fifth answer, with the same logic as the previous, is Licancabur volcano in Chile/Bolivia, which quite as close to the latitude of the perihelion but is significantly higher than Sairecabur.

What is the correct answer and why?

What place on Earth is closest to the Sun?

Answer 1

This is an interesting question, but it lacks a key factor that is crucial to the answer: TIME.

The point on Earth closest to the Sun varies through time, so the question can be asked about any moment in time, or over periods of time. Let's analyze the factors involved.

At any given moment in time, the point on Earth's surface that is closest to the Sun is what is called the “subsolar point”. This point corresponds to the point on the surface that intersects the imaginary line that connects the center of the Earth to the center of the Sun. In other words, the subsolar point correspond to the point on Earth surface where the sunlight hits the Earth perpendicular to the ground, therefore, a vertical object would project no shadow.

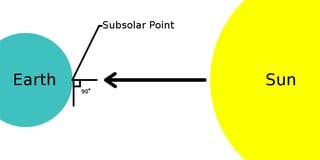
(image from Wikipedia: subsolar point)

The longitude of the subsolar point corresponds to that of the meridian experiencing solar noon. Over Greenwich (longitude 0°) that happens at the actual noon, and as the Earth rotates 15° every hour, that will happen one our later (at 13:00 h UTC) at longitude 15° W, two hours later (at 14:00 h UTC) at longitude 30° W, and so on. In general terms, you can use the following formula for the subsolar point longitude ($\text{SSP}_{\text{long}}$).

$\text{SSP}_{\text{long}} = \left(\text{UTC} -12\right)*15°$

This is a simplified formula, but accurate enough for our purpose. Let's take as an example the following date

July 20, 1969, at 20:17 UTC

In that moment, the longitude of the subsolar point was 124° 15' West:

$(20+(17/60)-12)*15°=124.25°=124°15'$

Finding the latitude of the subsolar point is a bit more complicated, we need to know the declination of the Sun. Declination is the equivalent of latitude for celestial coordinates. For that, use a formula, a table, or a online calculator like the NOAA Solar Position Calculator.

Just enter the date, and even that the location doesn't matter here, we need to select “Enter Lat/Long -->” to be allowed to enter the offset to UTC as 0, otherwise the time won't be interpreted as UTC time.

From there we can find that the solar declination for our example date is 20.58° (20° 34') which corresponds to the latitude of the subsolar point: 20° 34' North.

Therefore, on July 20, 1969, at 20:17 UTC, the subsolar point was at 20° 34' N, 124° 15' W, which is somewhere between Mexico and Hawaii. That was the point on Earth closest to the Sun at that moment.

Now, what would happen if there were a very tall mountain close to the subsolar point? Would that mountain be closer to the Sun?

The answer is: probably. It depends on how far and how much higher it is relative to the subsolar point.

We can do a quick calculation based on the following diagram (in this approximation we assume that Earth is spherical, that the sun is infinitely far away and other simplifications)

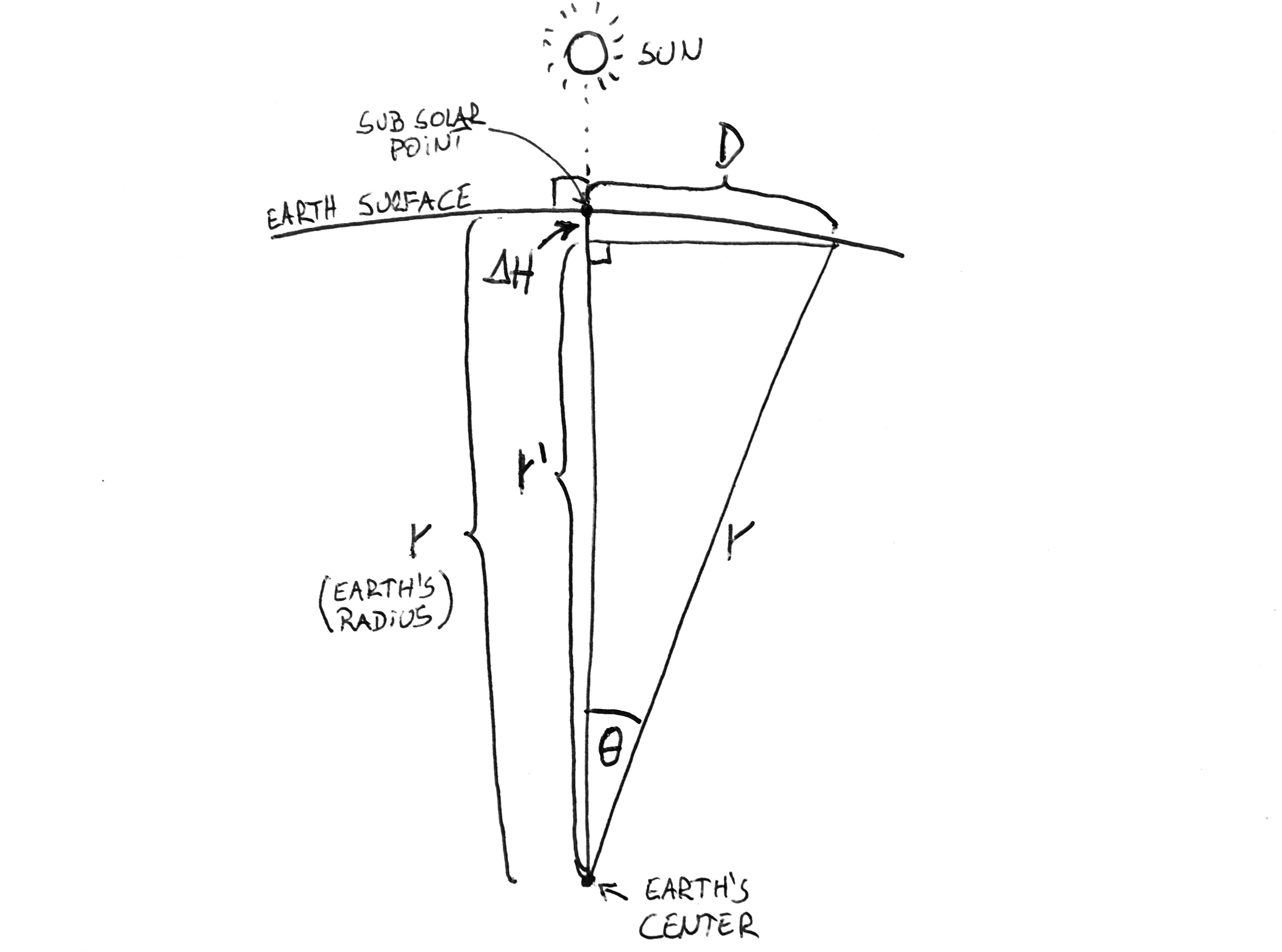
From there we have

$r-r'=\Delta H$

$D = r ~ \theta$ ($\theta$ in radians)

$\frac{r'}{r}=\cos(\theta)$

After some algebra you can write that the extra height $\Delta H$ needed to be as close to the Sun as the subsolar point is

$\Delta H = r \left(1-\cos\left(\frac{D}{r}\right)\right)$

Where $D$ is the distance and $r$ is Earth's radius (in this case makes sense to use the equatorial radius of 6378.1 km)

If we plot this equation we get the following

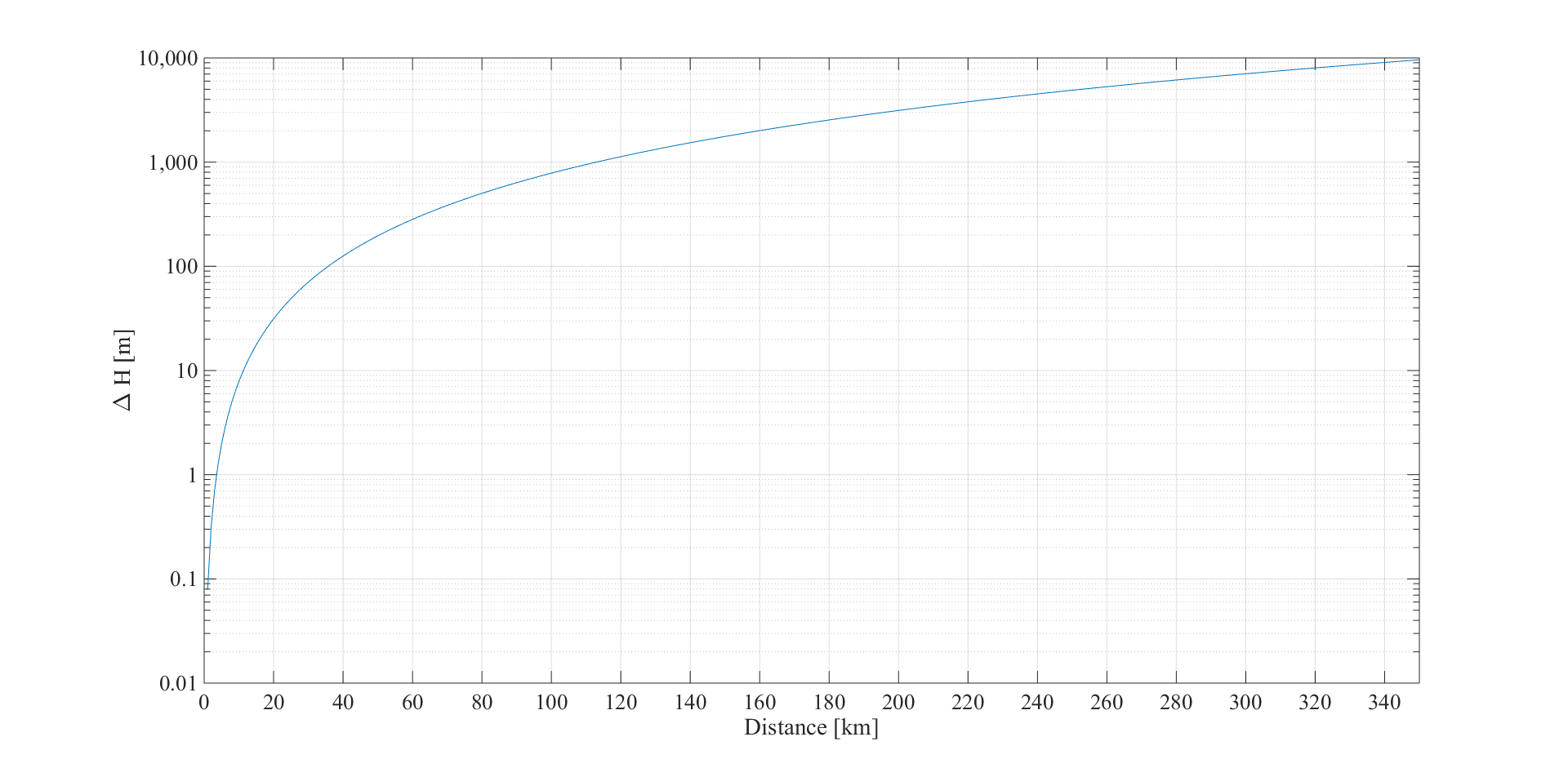
(the vertical axis is logarithmic)

We can see that around 10 km away from the subsolar point, ~10 meters are enough to be closer than it to the Sun. ~30 meters at 20 km, ~800 meters at 100 km, ~3,000 m at 200 km, and if you go further than 340 km, not even Mount Everest will get you closer to the Sun.

So, the closest point to the Sun will be whatever geographical feature that maximizes the value $\text{Altitude}-\Delta H$, where $\text{Altitude}$ is the altitude of the geographical feature. Let's call that point “proxisolar” point. I just made up that name, but it will be handy for the following discussion.

Now that we understand the basis to establish what is the closest point to the Sun at a given moment, we can tackle the question that probably most people meant when asking this question:

What is the point on Earth that gets closest to the Sun over a year?

The most important fact to keep in mind, is that the variations of the distance between the Earth and the Sun over the year dwarf any topographical feature and even the diameter of the Earth itself. Earth’s distance from the Sun (center-to-center) varies from 147,098,074 km at perihelion (closest) to 152,097,701 km at aphelion (most distant). Therefore, the difference is 5 million kilometers!.

The perihelion happens around January 4th, when the solar declination is about -23°, therefore, the latitude of the subsolar point is around 23° South. That rules out Chimborazo, Cayambe and Everest, because they are too far to be the “proxisolar” point. In contrast, Sairecabur (5,971 m at 22.72° S) and Licancabur (5,916m at 22.83° S) are reasonable contestants.

The problem is that the perihelion happens on different days of the year and at different times of the day every year, so the point that gets closest to the Sun on a given year is just the one that happen to be the “proxisolar point” at the time of the Perihelion.

People who argues that Sairecabur or Licancabur are the points that get closer to the Sun, are implicitly assuming that the distance Earth-Sun doesn't vary much during the day of the perihelion. Therefore, the extra elevation of these mountains allows them to get closer to the Sun during that day. Unfortunately, that assumption is completely wrong. Let's see why:

An approximation of the distance Earth-Sun can be obtained from the following formula

$d = \frac{a(1-e^2)}{1+e \cos\left(\text{days}\frac{360}{365.25}\right)}$

Where $a$ is the semi-major axis of Earth's orbit, $e$ is the eccentricity, and $\text{days}$ is the number of days elapsed since the perihelion. To see the simplifications behind this equation look here (note that the conversion factor 360/365.25 is erroneously inverted in that link, thanks @PM2Ring for spotting that).

If you solve the above equation for the perihelion and for one day before/after it, you will get that the difference is 358 km, and for half a day you get 89 km. Therefore, if the subsolar point happens to be on the opposite side of the Earth than, let's say, Licancabur volcano, this volcano would need to be 89 km higher that the subsolar point to get closer than it to the Sun that year. 89 kilometers!

Therefore, we can discard the idea that a given mountain could be the point that gets closer to the Sun on EVERY year.

If we plot the above equation with distances relative to the perihelion we get the following (using $a$ and $e$ from here)

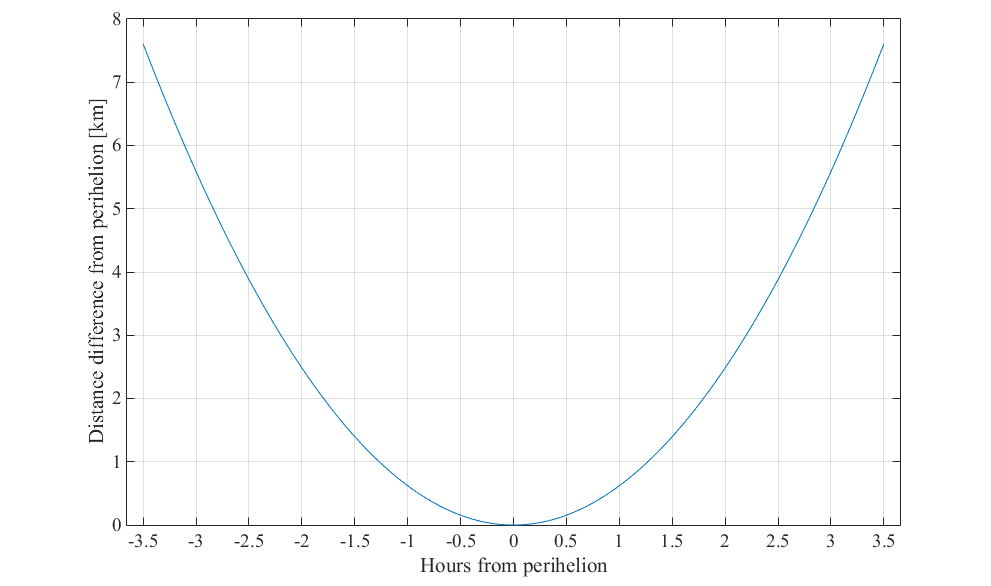
Here we can see, that if the perihelion happens a bit more than 3 hours before or after the solar noon at Licancabur, the ~6,000 m of elevation advantage would not be enough to get closer to the Sun than the subsolar point at the perihelion, even if such point is at sea level.

Note that three hours corresponds to 45° in Longitude, which at that approximate latitude corresponds to approximately 4,600 km.

Therefore, it can be argued that Licancabur is the point on Earth that has more chances to be the closest to the Sun in an arbitrary year. But in a given year, it might or might not be the closest depending on where the subsolar point is at the moment of the perihelion.

Finally, it is important to note that the distance Earth-Sun at the perihelion varies widely from year to year. If you look at this table of perihelions between years 2001 and 2100, you will see that perihelions often vary by several thousand of kilometers.

Therefore, for example between years 2001 and 2100, the closest perihelion by far is the perihelion of next year (2020), and it will happen when the subsolar point is in the middle of the Indian ocean, about 12,700 km away from Licancabur and Sairecabur volcanoes. Therefore, the point that will be closest to the Sun this century will be one in the middle of the Indian ocean about 320 km south of Rodrigues Island.

Said this, the question of which point on Earth will get closest to the Sun depends on the period of time on which it is considered. For each year, each century and any other arbitrary period of time, the answer will be different.

Answer 2

It is impossible to know. Solar flares can have more than 500,000 kilometers. So if we consider them part of the sun, the moment when the earth is closer to the sun can be very different from perihelion if a big flare happens, making much of what was discussed in other answers irrelevant.

Answer 3

The point on the surface of the Earth where the Sun is currently immediately overhead is called the Zenith Point. Its Latitude and Longitude correspond to the Declination and Greenwich Hour Angle of the Sun.

These data points can be approximated to any degree of accuracy and timeframe by a Fourier series of n terms. Accuracy sufficient for sextant work for the current decade can be achieved with a Fourier series of 7 terms). These terms are published in nautical almanacs.

Answer 4

Whichever spot on the surface of the Earth is experiencing Lahaina Noon, or would be if it wasn't cloudy, is at the subsolar point and pointed directly at the Sun, moreso than any other point on Earth at that moment. Of course, you could get closer to the sun by climbing higher.

If you were able to be at the summit of Chimborazo volcano in Ecuador (point furthest from the center of Earth) at Lahaina Noon at perihelion, that would be as close to the Sun as you can get and still be on the surface of the Earth. You'd probably have to be very lucky or extremely patient for that to happen, though.

Answer 5

There are some wonderful answers here, but I think a simplified plain English answer would be helpful. Barring any nearby mountains, and various foibles, the closest point to the sun at the June solstice is where it is midday on the tropic of Cancer. At the December solstice, it is where it is midday on the tropic of Capricorn. At equinox it is where it is midday on the equator. Other times of year it is a proportional point in between (no doubt described mathematically by the above equations).

Answer 6

At any one specific moment the subsolar point is the point on Earth that is closest to the Sun at that specific moment.

The subsolar point on a planet is the point at which its sun is perceived to be directly overhead (at the zenith);[1] that is, where the sun's rays strike the planet exactly perpendicular to its surface. It can also mean the point closest to the sun on an astronomical object, even though the sun might not be visible.

To an observer on a planet with an orientation and rotation similar to those of Earth, the subsolar point will appear to move westward, completing one circuit around the globe each day, approximately moving along the equator. However, it will also move north and south between the tropics over the course of a year, so it is spiraling like a helix.

The subsolar point contacts the Tropic of Cancer on the June solstice and the Tropic of Capricorn on the December solstice. The subsolar point crosses the Equator on the March and September equinoxes.

When the point passes through Hawaii, the only U.S. state in which this happens, it is known as Lahaina Noon.[2]

There is a minor exception to this rule.

The subsolar point can be defined as a mathematical point, so that someone could theoretically be standing with their body over the exact mathematical subsolar point and with their two legs and feet on either side of the exact mathematical subsolar point.

Thus a place that is close to the the exact mathematical subsolar point and at a higher altitude than it might be closer to the Sun than the exact mathematical subsolar point. The farther away from the exact mathematical subsolar point a place is the higher its altitude must be in order to be closer to the Sun than the exact mathematical subsolar point.

Earth has an polar radius of 6,356.8 kilometers and an equatorial radius of 6,378.1 kilometers, so a place at the equator is 21.3 kilometers farther from the center of the Earth than a place at one of the poles, not allowing for minor variations in the figure of the Earth and not allowing for altitude differences.

The subsolar point of earth is never farther north than the Tropic of Cancer and never farther south than the Tropic of Captricorn, and so in recent times is never North of about 23 degrees 26 minutes North Latitude or south of about 23 degrees 26 minutes South Latitude.

Of the points mentioned in the question Chimborazo and Cayambe Volcanoes in Ecuador, and Sairecabur and Licancabur Volcanoes in Chile, are within the tropics and thus can sometimes be the subsolar points, while Mount Everest is north of the Tropic of cancer and can never be the subsolar point.

If Earth as a radius of 6,356.8 to 6,378.1 kilometers, it must have diameter of 12,713.6 to 12,756.2 kilometers, and thus at any one moment the subsolar point, wherever it is at that moment, will be about 12,713.6 to 12,756.2 kilometers closer to the Sun than the point that is farthest from the Sun, wherever it is at that moment.

The semi-major axis of the orbit of the Earth is 149,598,023 kilometers, which can be taken as the average distance between the Earth and the Sun. Whenever the Earth is exactly 149,598,023 kilometers, the subsolar point will be about 12,713.6 to 12,756.2 kilometers closer to the Sun than the point that is farthest from the Sun.

So at any moment when Earth was exactly 149,598,023 kilometers from the Sun, and the subsolar point happens to be 12,756.2 kilometers closer to the Sun than the point on Earth farthest from the Sun, the difference will be 1 in 11,727.475, or 0.0000852 of the distance between the Earth and the Sun.

The perihelion distance between Earth and the Sun is 147,095,000 kilometers while the aphelion distance between Earth and the Sun is 152,100,000 kilometers, a difference of 5,005,000 kilometers. Since it takes earth about half a year, or about 182.625 days, to go from Aphelion to perihelion, the distance between Earth and the Sun changes by an average of about 27,405.886 kilometers per day.

Thus any point on Earth, even the point on Earth that is farthest from the Sun, at the exact moment of perihelion should still be closer to the Sun than any point on Earth, even the subsolar point, on any other day of the year.

What about astronauts in orbit, bound by gravity to the Earth? Do their capsules and space stations count? Whether or not space capsules and space stations count as part of Earth, some astronaut in Earth orbit might have been closer to the Sun and another astronaut might in earth orbit have been farther from the Sun than any human in history.

And what about Apollo astronauts who traveled to the Moon and orbited or passed beyond it? Does the Moon count as a place on Earth since it is bound to Earth by gravity? And even if not, some Apollo astronaut or astronauts must have been farther from Earth than any other humans.

The Apollo missions all lasted for at least a quarter of a lunar orbit around the Earth. The landings on the Moon were all on the near side of the Moon that faces the Earth, and all during the Lunar day, which means that the near side of the Moon must have been facing the Sun and thus farther from the Sun than Earth during the missions.

Earth is at perihelion, closest to the Sun around January 4, and at aphelion, farthest from the Sun, around July 4.

So of the manned missions that reached Lunar orbit, Apollo 11, July 1624, 1969, and Apollo 15, July 26-August 7, 1971, were closest to aphelion about July 4, and so their astronauts might have traveled farther from the Sun than any other humans, depending on where the Moon was during its orbit.

And Apollo 8, December 21-27 1968, Apollo 17, December 7-19 1972, and Apollo 14, January 31-February 9, 1971, were closed to perihelion about January 4. And so the astronauts in one of those missions might have come closest to the Sun of all humans. But I doubt that. Probably some astronaut(s) who orbited the Earth several times on a January 4 passed close to a point above the subsolar point at a time very close to perihelion and came closed to the Sun - or least far from it - of all humans so far.

Answer 7

The closest point on the Earth to the Sun needs be a subsolar point on a monumental tropical summit, on January 4th during a micromoon, when the the Moon is maximally out, and the Earth is maximally inside our shared Earth/Moon orbit.